NO.PZ2017092702000079
问题如下:
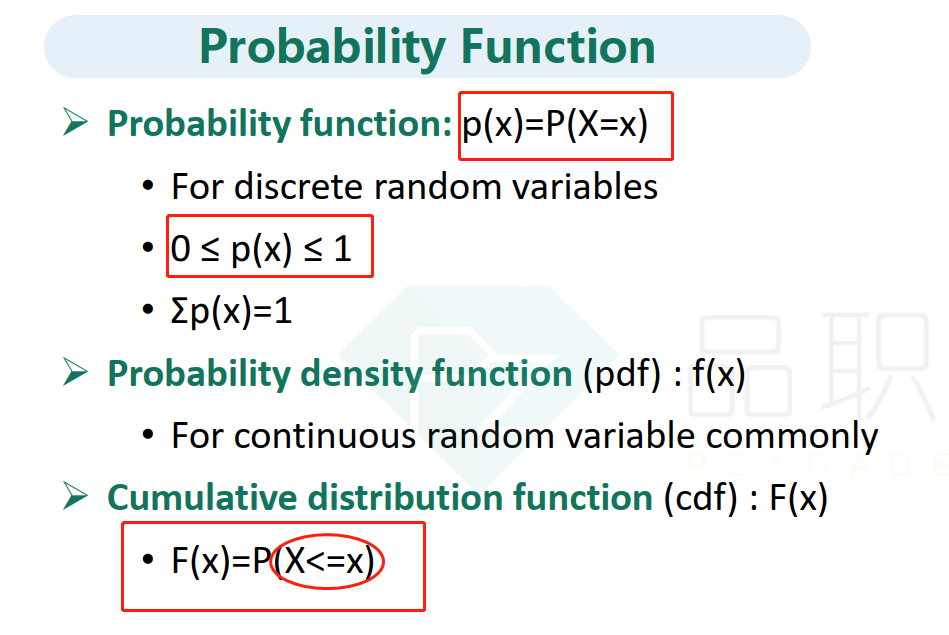
The value of the cumulative distribution function F(x), where x is a particular outcome, for a discrete uniform distribution:
选项:
A. sums to 1.
B. lies between 0 and 1.
C. decreases as x increases.
解释:
B is correct.
The value of the cumulative distribution function lies between 0 and 1 for any x: 0 ≤ F(x) ≤ 1.
对于任何 x值,累积分布函数的值介于 0 和 1 之间:0 ≤ F(x) ≤ 1。
该题问的是离散均匀变量,A选项为什么不对