NO.PZ202109130200004502
问题如下:
Based on Exhibit 1, Olabudo should calculate a prediction interval for the actual US CPI closest to:
选项:
A.2.7506 to 2.7544
B.2.7521 to 2.7529
C.2.7981 to 2.8019
解释:
A is correct. The forecast interval for inflation is calculated in three steps:
Step 1. Make the prediction given the US CPI forecast of 2.8:
Y^=b0+b1X=0.0001+(0.9830×2.8)=2.7525
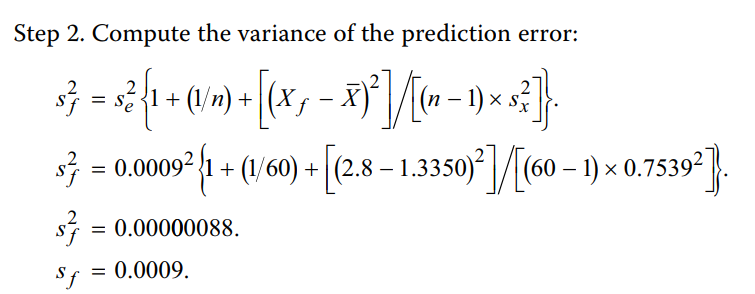
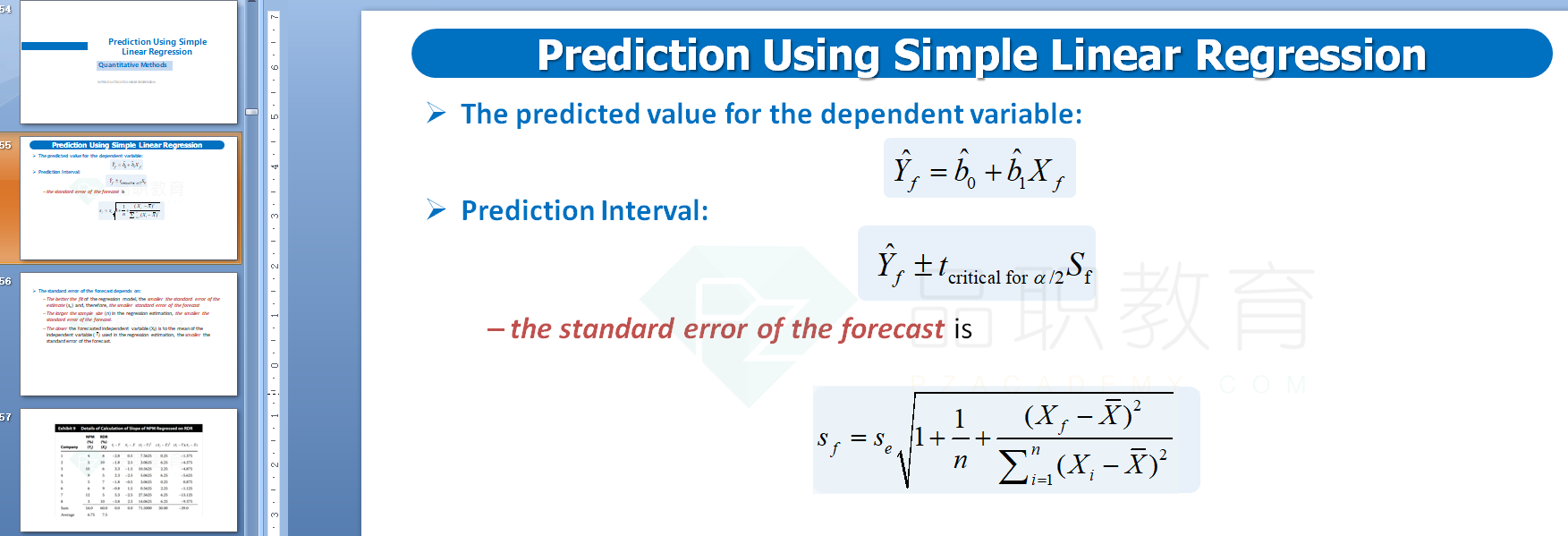
Step 2. Compute the variance of the prediction error:
sf2=se2{1+(1/n)+[(Xf−Xˉ)2]/[(n−1)×sx2]}
sf2=0.0009e2{1+(1/60)+[(2.8−1.3350)2]/[(60−1)×0.75392]}
sf2=0.00000088
sf=0.0009
Step 3. Compute the prediction interval:
Y^±tc×sf
2.7525±(2.0 x 0.0009)
Lower bound: 2.7525 - (2.0 x 0.0009) = 2.7506.
Upper bound: 2.7525 + (2.0 x 0.0009) = 2.7544.
So, given the US CPI forecast of 2.8, the 95% prediction interval is 2.7506 to 2.7544.
老师,可以帮忙讲一下第二步骤吗?,没看懂答案