没有明白答案中得1101是从那里来得，问题8中的fair value 不是1101呀，应该是1098.1385？请问是不是我那里理解的不对。麻烦能不能把计算过程写一遍。谢谢-有问必答-品职教育专注CFA ESG FRM CPA 考研等财经培训课程

没有明白答案中得1101是从那里来得，问题8中的fair value 不是1101呀，应该是1098.1385？请问是不是我那里理解的不对。麻烦能不能把计算过程写一遍。谢谢

* 问题详情，请查看题干

NO.PZ201812310200000109

问题如下：

Daniela Ibarra is a senior analyst in the fixed-income department of a large wealth management firm. Marten Koning is a junior analyst in the same department, and David Lok is a member of the credit research team.

The firm invests in a variety of bonds. Ibarra is presently analyzing a set of bonds with some similar characteristics, such as four years until maturity and a par value of €1,000. Exhibit 1 includes details of these bonds.

Ibarra asks Koning to assist her with analyzing the bonds. She wants him to perform the analysis with the assumptions that there is no interest rate volatility and that the government bond yield curve is flat at 3%.

Ibarra performs the analysis assuming an upward-sloping yield curve and volatile interest rates. Exhibit 2 provides the data on annual payment benchmark government bonds. She uses this data to construct a binomial interest rate tree (shown in Exhibit 3) based on an assumption of future interest rate volatility of 20%.

Exhibit 3. One-Year Binomial Interest Rate Tree for 20% Volatility

Answer the first five questions (1–4) based on the assumptions made by Marten Koning, the junior analyst. Answer questions (8–12) based on the assumptions made by Daniela Ibarra, the senior analyst.

Note: All calculations in this problem set are carried out on spreadsheets to preserve precision. The rounded results are reported in the solutions.

Note: You may use the Exhibit 7 from the reading. Here is the Exhibit 7.

Ibarra wants to know the credit spread of bond B2 over a theoretical comparable-maturity government bond with the same coupon rate as this bond. The foregoing credit spread is closest to:

选项：

108 bps.

101 bps.

225 bps.

解释：

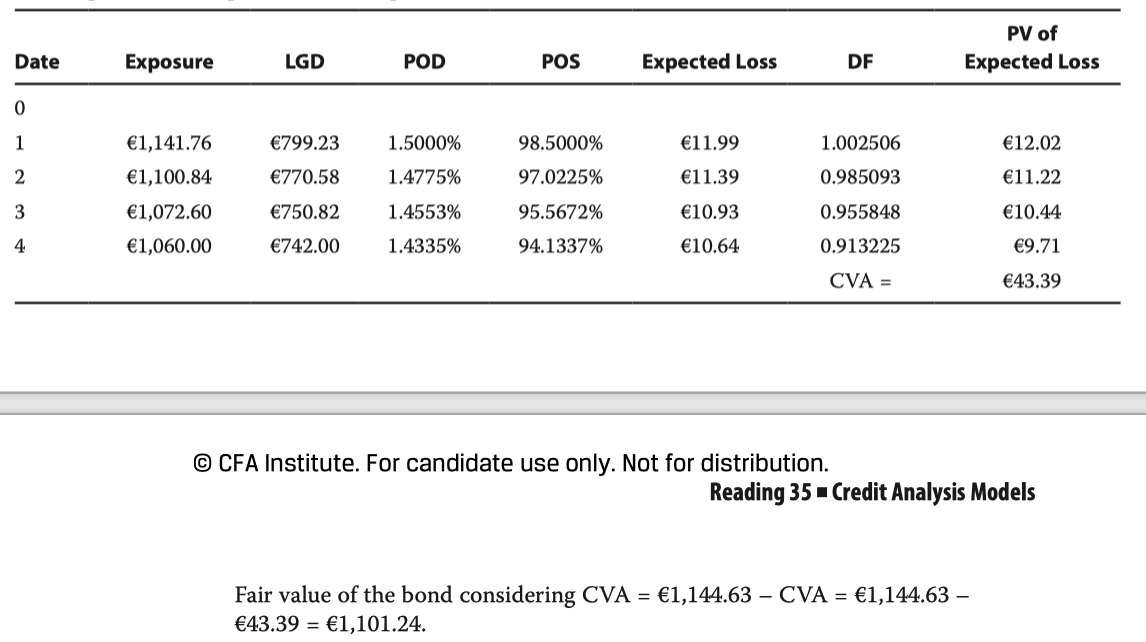
A is correct. The corporate bond’s fair value is computed in the solution to Question 8 as €1,101.24 The YTM can be obtained by solving the following equation for IRR:

$1101.24 = \frac{60}{1 + I R R} + \frac{60}{{(1 + I R R)}^{2}} + \frac{60}{{(1 + I R R)}^{3}} + \frac{1060}{{(1 + I R R)}^{4}}$

The solution to this equation is 3.26%.

Valuation of a four-year, 6% coupon bond under no default (VND) is computed in the solution to Question 8 as 1,144.63. So, the YTM of a theoretical comparable-maturity government bond with the same coupon rate as the corporate bond B2 can be obtained by solving the following equation for IRR:

$1144.63 = \frac{60}{1 + I R R} + \frac{60}{{(1 + I R R)}^{2}} + \frac{60}{{(1 + I R R)}^{3}} + \frac{1060}{{(1 + I R R)}^{4}}$

The solution to this equation is 2.18%. So, the credit spread that the analyst wants to compute is 3.26% – 2.18% = 1.08%, or 108 bps.

B is incorrect, because that is the spread over the four-year government par bond that has a YTM of 2.25% in Exhibit 2: 3.26% – 2.25% = 1.01%, or 101 bps. Although this spread is commonly used in practice, the analyst is interested in finding the spread over a theoretical 6% coupon government bond.

C is incorrect, because that is the YTM of the coupon four-year government bond in Exhibit 2.

没有明白答案中得1101是从那里来得，问题8中的fair value 不是1101呀，应该是1098.1385？请问是不是我那里理解的不对。麻烦能不能把计算过程写一遍。谢谢

1 个答案

1

1

589

相关问题