NO.PZ201712110200000401
问题如下:
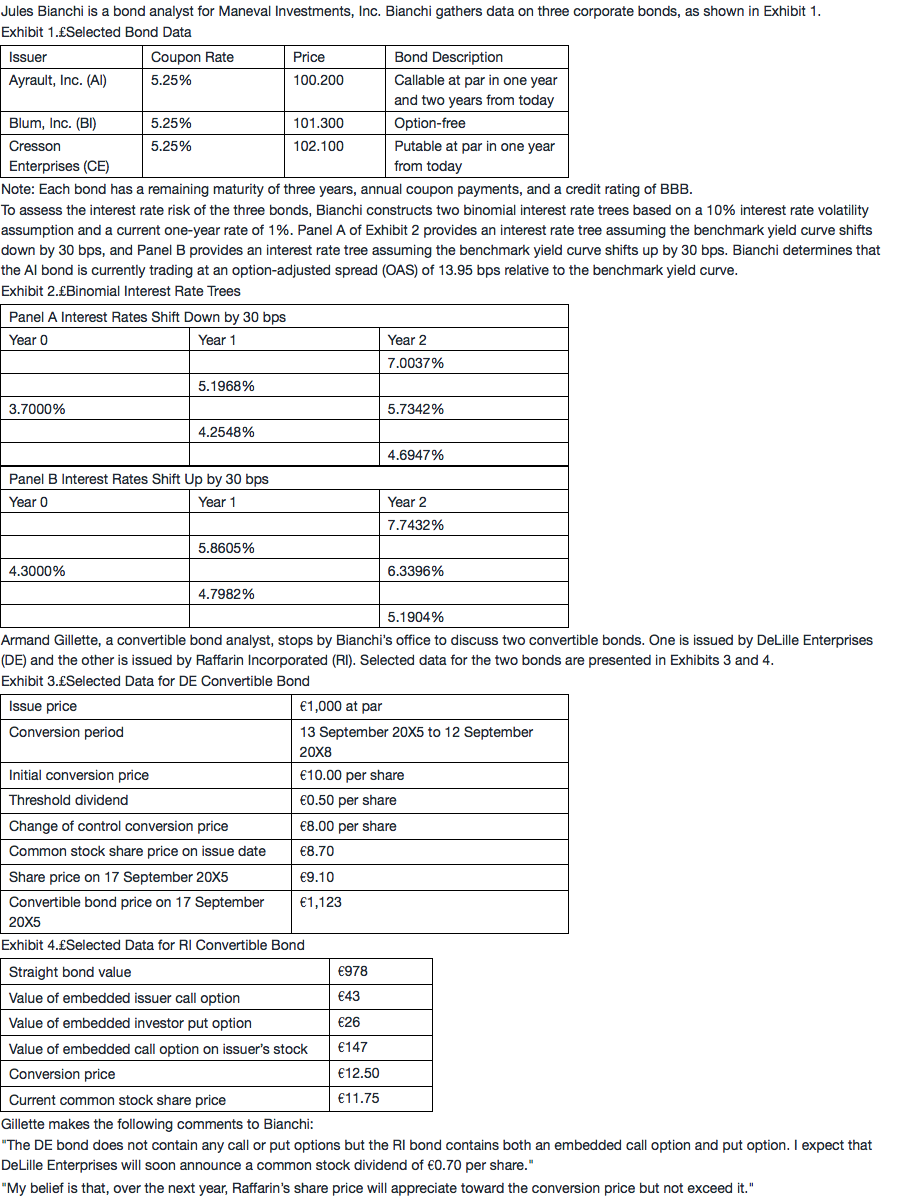
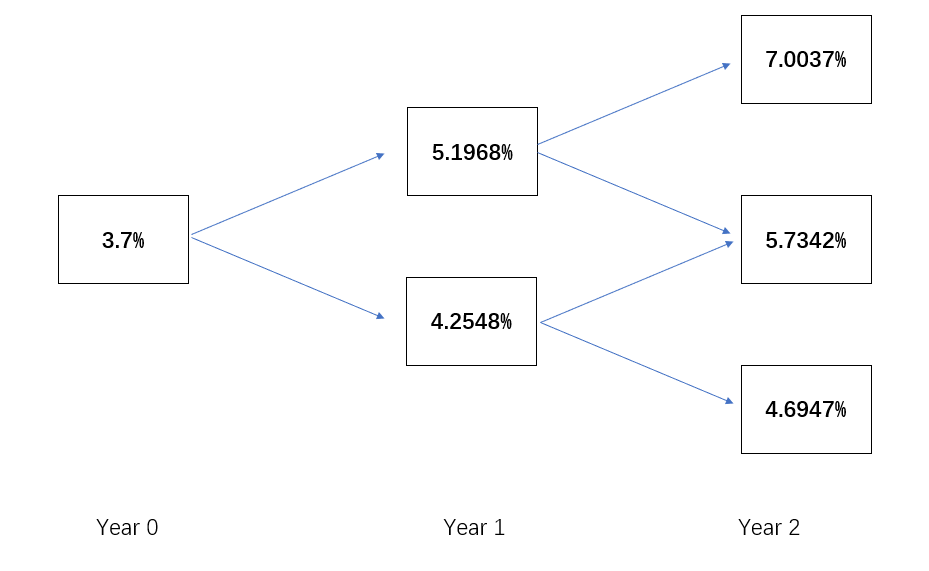
Based on Exhibits 1 and 2, the effective duration for the AI bond is closest to:
选项:
A.1.98.
B.2.15.
C.2.73.
解释:
B is correct.
The AI bond’s value if interest rates shift down by 30 bps (PV–) is 100.78. The AI bond’s value if interest rates shift up by 30 bps (PV+) is 99.487.
Effective duration=[(PV-)-(PV+)]/[2× (ΔCurve) × (PV0)]= (100.780 - 99.487)/ (2 × 0.003 × 100.200)=2.15
请问在计算V-和V+的时候我们不考虑会被Call会吗?不需要做判断吗?谢谢