我来问一道官网上的题目,case和题目如下。这道题对delta的描述错在哪里?gamma的定义是delta的变化(价格变化百分比的二阶倒),“changes in volatility”怎么理解?谢谢!
Applegate Capital Management Case Scenario
Pari Patel is a senior portfolio manager at Applegate Capital Management, an institutional hedge fund manager in San Francisco that offers a variety of strategies. Patel oversees the management of the Applegate Hedged Alpha Fund, a mutual fund with the objective of providing capital appreciation while minimizing downside risk. Justin Flaherty is a junior analyst on Patel’s team.
Patel works closely with Applegate’s risk and compliance teams to ensure he understands the inherent risks in the portfolio. Each morning, Patel receives a risk report with a number of metrics to help him assess portfolio exposures and risks.
Patel is reviewing the daily risk report with Flaherty to discuss why value at risk (VaR) is an important measure of risk. Flaherty makes the following three statements regarding VaR:
Statement 1VaR measures the minimum expected loss, rather than the maximum expected loss, in a portfolio.
Statement 2VaR is used to estimate portfolio losses that are likely to occur at a fixed point in time, rather than over a period of time.
Statement 3VaR measures portfolio volatility in percentage terms.
Patel replies to Flaherty’s statements by focusing on specific aspects of VaR: “Applegate’s process begins with a risk decomposition of the portfolio holdings, typically assumes normal distribution of risk factors, and then uses the expected return and standard deviation for each risk factor to estimate the VaR. The VaR threshold is converted to a z-distribution. We then calculate the expected return and volatility of the portfolio and adjust to the desired time interval. Finally, we can obtain VaR and convert it into a dollar amount by multiplying by the portfolio value.”
In reviewing the VaR report, Patel would like more insight on the average loss that would occur if the VaR cutoff is extended and asks Flaherty to contact the risk team to improve the reporting metrics. After discussing with his colleague on the risk team, Flaherty responds that going forward they will add conditional VaR, incremental VaR, and marginal VaR to the report.
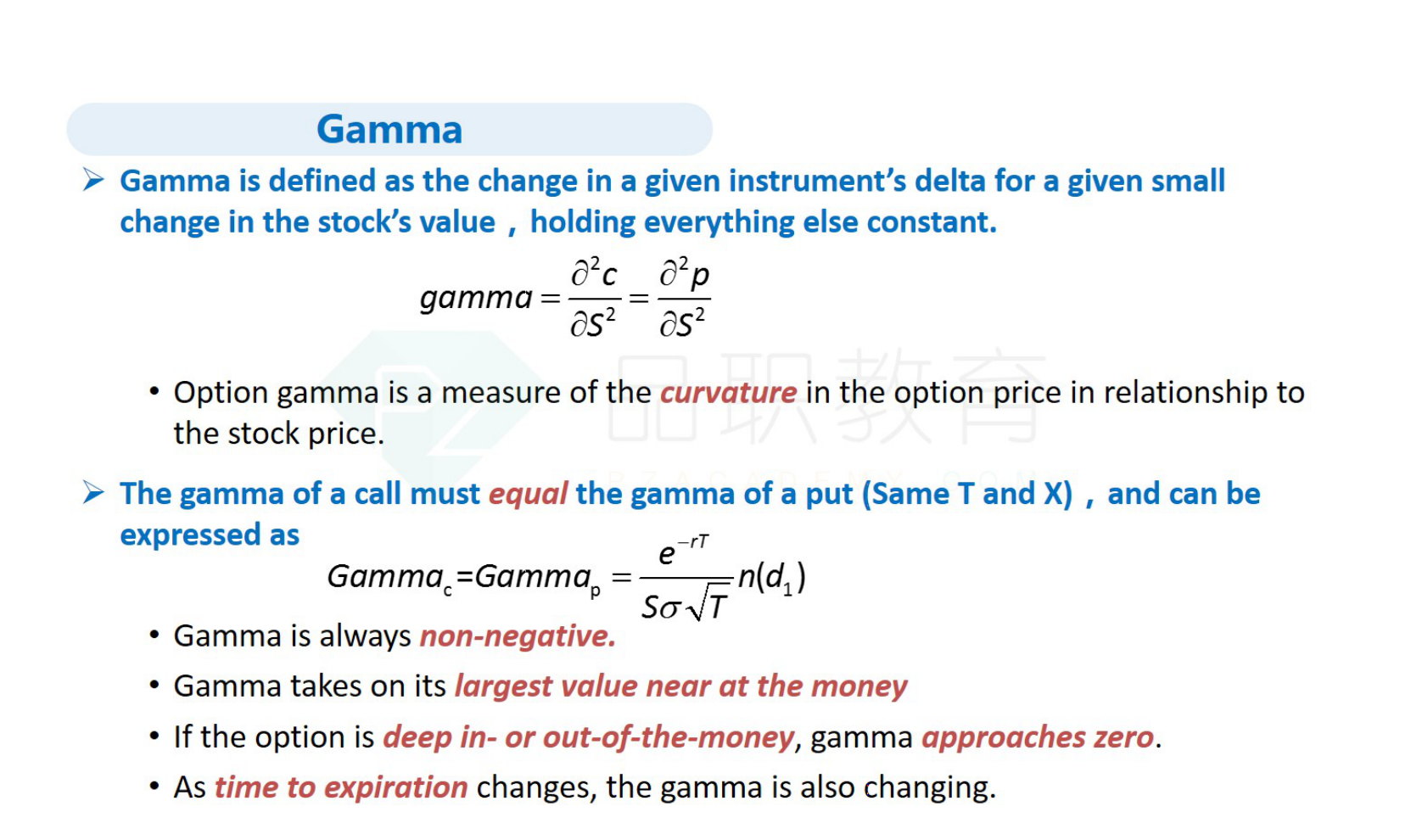
In addition to VaR, the risk report includes other measures that assist Patel in better understanding portfolio sensitivities. Patel uses option strategies within the fund to manage exposures. Patel asks Flaherty to review sensitivity risk measures to better understand the option exposure within the fund. Flaherty uses gamma to understand how sensitive option prices are to an increase in volatility of the underlying stock and delta to determine how large moves in the value of the fund positions will affect associated option values.
Marcus Thompson oversees risk management at Applegate on a firm-wide basis. Because of the use of leverage, Thompson pays particular attention to risk measures to understand the sources and uses of cash and the risk of hitting leverage limits and facing margin calls. He uses a number of metrics in his risk dashboard and communicates to managers and firm leadership when actions need to be taken to mitigate risk.
Thompson frequently reviews Applegate’s strategies to identify those with greater-than-expected tail risk. Doing so helps him understand potential capital allocation requirements for meeting tail risk losses. He is asked to review the following three newly proposed fund strategies and to assess potential tail risk and capital requirements:
- Strategy 1: Long–short equity fund targeting an equity market beta of 0.2 based on fundamental long and short equity research
- Strategy 2: Long-only option strategy that uses technical analysis and momentum to trade call and put options on a short-term basis
- Strategy 3: Alternative income strategy focused on long credit positions combined with selling insurance and writing options to generate premium income
Is Flaherty most likely correct in his use of sensitivity measures to assess the impact of the option positions in the fund?
A.Yes
B.No, with regard to delta
C.No, with regard to gamma
Solution
C is correct. Gamma is the measure of the change in value of the delta (second derivative) relative to the change in value of the underlying and also does not address changes in volatility.
Vega is expressed as the change in the value of an option over the change in volatility of the underlying.
A is incorrect. Flaherty is incorrect with regard to the discussion of gamma.
B is incorrect. Delta is the measure of the change in value of the option relative to the change in value of the underlying and does not address changes in volatility.
Measuring and Managing Market Risk Learning Outcome
- Describe the use of sensitivity risk measures and scenario risk measures



