NO.PZ2015120601000008
问题如下:
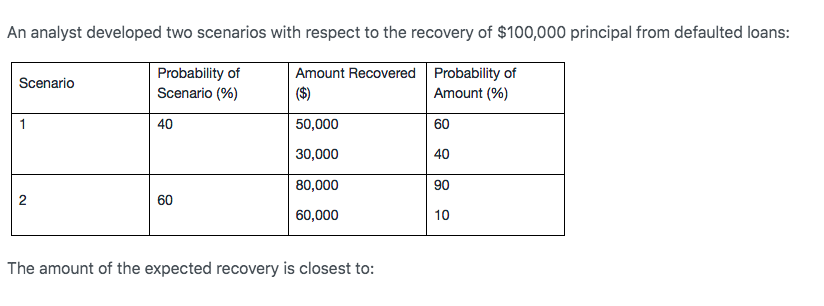
An analyst developed two scenarios with respect to the recovery of $100,000 principal from defaulted loans:

The amount of the expected recovery is closest to:
选项:
A.$36,400.
B.$55,600.
C.$63,600.
解释:
C is correct.
If Scenario 1 occurs, the expected recovery is 60% ($50,000) + 40% ($30,000) = $42,000, and if Scenario 2 occurs, the expected recovery is 90% ($80,000) + 10%($60,000) = $78,000.
Weighting by the probability of each scenario, the expected recovery is 40%($42,000) + 60%($78,000) = $63,600.
Alternatively, first calculating the probability of each amount occurring, the expected recovery is (40%)(60%)($50,000) + (40%)(40%)($30,000) + (60%)(90%)($80,000) + (60%)(10%)($60,000) = $63,600.
这道题考察加权平均计算均值(expected recovery),权重为概率。
①首先算出scenario 1中的加权平均值为50,000×60%+30,000×40%=42,000;
同理scenario 2中加权平均值为80,000×90%+60,000×10%=78,000.
②然后再将scenario1 &2做加权平均,
42,000×40%+78,000×60%=63,600,选择B选项。
这里搞不太清prob of amount (%)是不是乘以scenario后还是乘以scenario前的数字,如果这里是乘以scenario前的数字。那为什么视频中关于eps 那道题求E(EPS)直接乘以EPS?而没有再乘以probability of recession 25%? 所以视频中there is 25% of probabilty that EPS is $2 and there is 75% of probabilty that EPS is $4 中的25% and 75%代表的是已经乘以recession probability 0.25后的数字吗?还是仅仅代表乘recession probability以前的数字?如果同样如这道题是代表乘以scenario前的数字,为什么 E(EPS)不等于25%*2*25%+75%*4*25%?




