NO.PZ2016031001000089
问题如下:
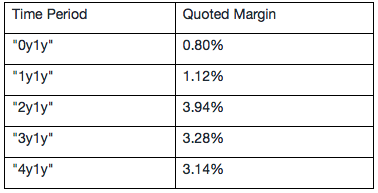
All rates are annual rates stated for a periodicity of one (effective annual rates).
The value per 100 of par value of a two-year, 3.5% coupon bond, with interest payments paid annually, is closest to:
选项:
A.101.58.
B.105.01.
C.105.82.
解释:
B is correct.
The value per 100 of par value is closest to105.01. Using the forward curve, the bond price is calculated as follows:

这道题直接用计算器算出和答案不同为107.3,为何之前讲过的用spot-rate求price计算器可以直接算出?




