问一道题：NO.PZ2019070101000020

问题如下：

An analyst wants to calculate the risk-neutral probability of an up-move for a three-month European call option on ABC stock, he has collected below information: continuously compounded risk-free rate is 4%, ABC stock pays a continuous dividend yield of 2%, and ABC has an annual standard deviation of 8%. Which of the following is correct?

选项：

0.55.

0.62.

0.79.

0.86.

解释：

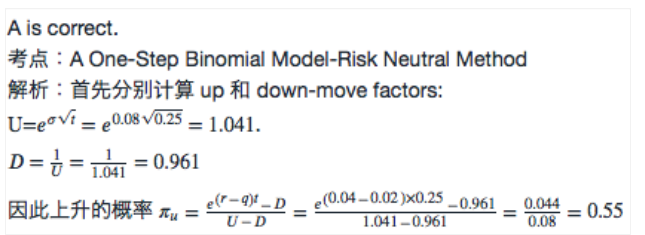
A is correct.

考点：A One-Step Binomial Model-Risk Neutral Method

解析：首先分别计算 up 和 down-move factors:

$U= e^{σ \sqrt{t}} = e^{0.08 \sqrt{0.25}} = 1.041$ .

$D = \frac{1}{U} = \frac{1}{1.041} = 0.961$

因此上升的概率 $π_{u} = \frac{e^{(r - q) t} - D}{U - D} = \frac{e^{(0.04 - 0.02)\times0.25} - 0.961}{1.041 - 0.961} = \frac{0.044}{0.08} = 0.55$

请问这是在课件哪一部分另答案好像显示不全

添加评论

1 个答案

已采纳答案

orange品职答疑助手 · 2020年03月02日

同学你好，这是二叉树部分，带股利分红时算上涨概率的考点。答案显示的有点问题，不好意思，我们会尽快修正~

当标的资产支付连续收益率为q的红利时，在风险中性条件下，证券价格的增长率，就由之前的r，变为了r-q，因此之前的e^(r*T)=pu+(1-p)*d，就应该变成 e^[(r-q)*T]=pu+(1-p)*d。之后就跟之前的步骤一样了。

期权带分红的情况，其实是类似的，就像我们平常算delta时一样，带有分红的期权delta是N(d1)*e^(-qT)。

添加评论

1
回答
0
关注
437
浏览

我要回答关注问题

相关问题

NO.PZ2019070101000020问题如下analyst wants to calculate the risk-neutrprobability of up-move for a three-month Europecall option on Astock, he hcollectebelow information: continuously compounrisk-free rate is 4%, Astopays a continuous vinyielof 2%, anAhannustanrviation of 8%. Whiof the following is correct? A.0.55.B.0.62.C.0.79.0.86.A is correct.考点A One-Step BinomiMol-Risk NeutrMetho析首先分别计算 up 和 wn-move factors:U=eσt=e0.080.25=1.041U=e^{\sigma\sqrt t}=e^{0.08\sqrt{0.25}}=1.041U=eσt=e0.080.25=1.0411U=11.041=0.961\frac1U=\frac1{1.041}=0.961U1=1.0411=0.961因此上升的概率 πu=e(r−q)t−−e(0.04−0.02)×0.25−0.9611.041−0.961=0.0440.08=0.55\pi_u=\frac{e^{(r-q)t}-{U-=\frac{e^{(0.04-0.02)\times0.25}-0.961}{1.041-0.961}=\frac{0.044}{0.08}=0.55πu=U−(r−q)t−=1.041−0.961e(0.04−0.02)×0.25−0.961=0.080.044=0.55老师好，这里的r是年化利率？也就是如果是1个月，3个月等这种情况，不用去年化？

2024-06-27 10:57 1 · 回答

请问为什么这个T=三个月=0.25在运算中要开根号呀

2020-05-26 06:12 1 · 回答

请问上升的概率中为什么要剔除分红的部分，道理上能理解，但因为之前课程只有讲不分红的情况。老师这部分公式的推导能否提供一下呢？

2019-10-13 00:14 1 · 回答

问一道题：NO.PZ2019070101000020

1 个答案

1

0

437

相关问题