问题如下:
The capital structure of HighGear Corporation consists of two parts: one five- year zero-coupon bond with a face value of $100 million and the rest is equity. The current market value of the firm’s assets is $130 million and the expected rate of change of the firm’s value is 25%. The firm’s assets have an annual volatility of 30%. Assume that firm value is lognormally distributed, with constant volatility. The firm’s risk management division estimates the distance to default using the Merton model, or Given the distance to default, the estimated default probability is
选项:
A.2.74%
B.12.78%
C.12.79%
D.30.56%
解释:
ANSWER: A
We compute . The PD is then .
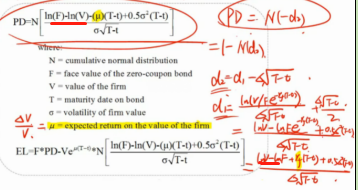
在公式里到底是LN(V/F)还是LN(F/V)
不太懂,老师写的是V/F,这道题还有讲义都是F/V
这样子就会算错啊



