问一道题：NO.PZ201902210100000110

问题如下：

The weekly volatility of Winslow’s portfolio is closest to:

选项：

0.56%.

0.80%.

0.63%

解释：

B is correct.

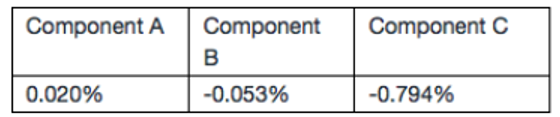
As mentioned previously, the three yield curve movements are uncorrelated, so the effect of each composite scenario is the sum of the contributions of the individual components. The following tables summarize the effects of all eight composite scenarios.

The mean for Winslow’s portfolio is zero because the returns for the eight composite scenarios sum to zero. To compute the weekly volatility (standard deviation), square and sum the returns, divide by 8, and take the square root. Note that the eight returns are actually four pairs of plus/minus the same number. So, the weekly volatility for Winslow’s portfolio is:

$\frac{\sqrt{(2\times {0.8270}^2)+(2\times {0.7210}^2)+(2\times {0.8670}^2)+(2\times {0.7610}^2)}}{8}=0.80\%$

A is incorrect. This answer results from squaring and summing four of the returns, dividing by 8, then taking the square root. This incorrectly accounts for the effects of only four of the eight composite scenarios.

$\frac{\sqrt{{0.8270}^2+{0.7210}^2+{0.8670}^2+{0.7610}^2}}{8}=0.56\%$

C is incorrect. This answer results from squaring and summing the returns, dividing by 8, but then failing to take the square root.

$\frac{(2\times {0.8270}^2)+(2\times {0.7210}^2)+(2\times {0.8670}^2)+(2\times {0.7610}^2)}{8}=0.63\%$

为什么要这么计算啊？