月之流离 · 2020年01月15日
问题如下：
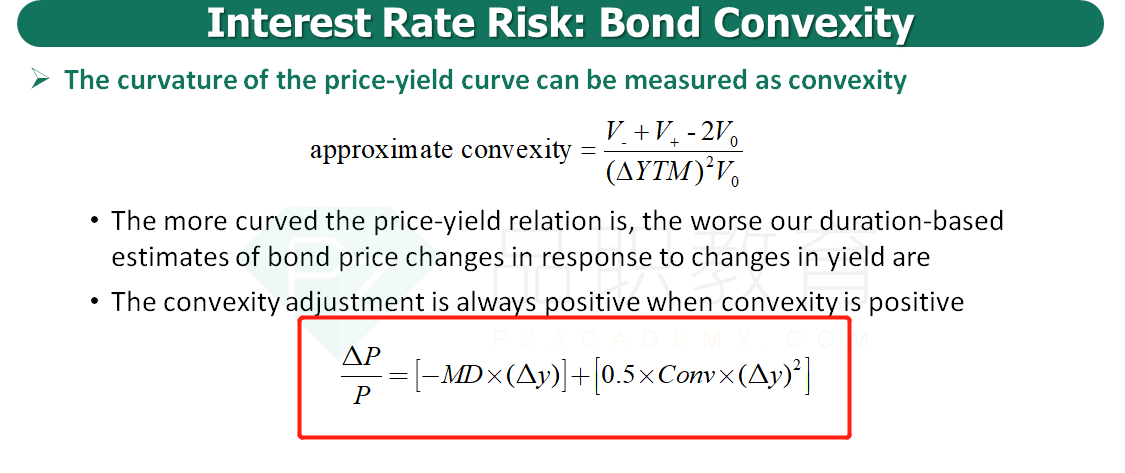
A bond has an annual modified duration of 7.140 and annual convexity of 66.200. The bond’s yield-to-maturity is expected to increase by 50 basis points. The expected percentage price change is closest to:
选项：
A.–3.40%.
B.–3.49%.
C.–3.57%.
解释：
B is correct.
The expected percentage price change is closest to −3.49%. The convexity adjusted percentage price change for a bond given a change in the yield-to-maturity is estimated by:
= -0.034873 or -3.49%
看不懂？这题为什么这样计算？
NO.PZ2016031001000128 问题如下 A bonhannumofieration of 7.140 anannuconvexity of 66.200. The bons yielto-maturity is expecteto increase 50 basis points. The expectepercentage prichange is closest to: A.–3.40%. B.–3.49%. C.–3.57%. B is correct.The expectepercentage prichange is closest to −3.49%. The convexity austepercentage prichange for a bongiven a change in the yielto-maturity is estimateby:%ΔPVFull≈[−AnnMour×ΔYiel+0.5×AnnConverxity×(ΔYiel^2%ΔPVFull≈[−7.14×0.005]+0.5×66.2×(0.005)^2= -0.034873 or -3.49%考点ration convexity解析这道题考察的是综合考虑ration和convexity对债券价格的影响。题干中利率上升50bps，即△yiel= 0.5% = 0.005，ration=7.14，Convexity=66.2。△P/P= -ration × △y+0.5 × convexity × (△y)2 = -7.14 × 0.5%+0.5 × 66.2 × (0.5%)2 = -3.49%故B正确。 为啥我用这个公式算出来的答案永远不一样-7.14x0.5%+0.5x66.2x（0.5%^2)第一个式子算出来是-0.0357第二个是0.000828两个相加也是正数啊
NO.PZ2016031001000128 按照解析的公式算出来是-0.04705吧？
你好，請問問這條題目是不是計算數字錯了？M該是7.140，所以算出來的答案應該是0.036528呀
这题有问题吧？解析按照公式计算出来的数字不对吧？
