问题如下图:

选项:
A. 
B. 
C. 
解释:
 老师您好,这道题要么失败一次,要么失败0次,那应该是0.7的0次方和0.7的1次方?而为什么是0.3?
老师您好,这道题要么失败一次,要么失败0次,那应该是0.7的0次方和0.7的1次方?而为什么是0.3?
星星_品职助教 · 2020年01月01日
同学你好,
这道题是二项分布的一个考点。题干中问没有完成rise more than 1 time的概率,就相当于仅成功(rise)了1次或者0次。
可以参照一下二项分布的公式,二项分布公式需要从“成功”概率角度出发,而不是从失败的概率角度。这里的成功概率就是rise的概率30%。
以仅成功(rise)一次为例。相当于同时满足两个条件:1. 三年中有一年成功; 2. 三年中的另外两年是失败(not rise)。所以概率不仅要包括成功那一次的0.3,还要包括另外两年都要失败的概率0.7的平方,才能满足“仅”成功一次的概率。所以是3C1*(0.3^1)*(0.7^2)=0.441.
同理,仅成功0次(全失败)的概率是3C0*(0.3^0)*(0.7)^3=0.343.
所以没有完成目标的总概率就是以上两者相加。
新年快乐~
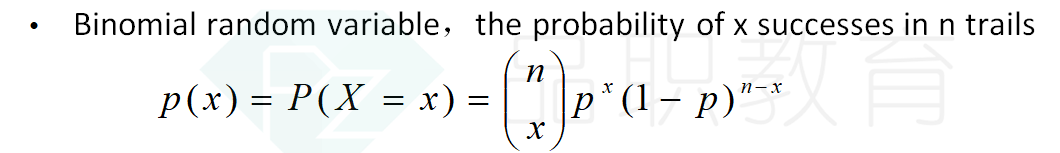
NO.PZ2018062016000083 问题如下 The stoof Acompany ha 30% probability to rise every year, assume thevery annutriis inpennt from eaother. If the stomeets the goof rising more th1 time in the next 3 years, whis the probability thit fails to meet the goal? A.0.343 B.0.216 C.0.784 C is correct. Baseon the corresponng formula:p(x)=P(X=x)=(nx)px(1−p)n−xp{(x)}=P{(X=x)}={(\begin{array}{c}n\\x\enarray})}p^x{(1-p)}^{n-x}p(x)=P(X=x)=(nx)px(1−p)n−x, n = 3 anp = 0.30.p(1)=3!(3−1)!1!×0.31(1−0.3)2=(3)(0.3)(0.49)=0.441p(1)=\frac{3!}{(3-1)!1!}\times0.3^1(1-0.3)^2=\left(3\right)\left(0.3\right)\left(0.49\right)=0.441p(1)=(3−1)!1!3!×0.31(1−0.3)2=(3)(0.3)(0.49)=0.441p(0)=3!(3−0)!0!×0.30(1−0.3)3=(1)(1)(0.343)=0.343⋅p(0)=\frac{3!}{(3-0)!0!}\times0.3^0(1-0.3)^3=\left(1\right)\left(1\right)\left(0.343\right)=0.343\ctp(0)=(3−0)!0!3!×0.30(1−0.3)3=(1)(1)(0.343)=0.343⋅The requireprobability is: p(1) + p(0) = 0.441 + 0.343 = 0.784 老师 能不能麻烦具体讲解一下这道题的思路 是要运用到nCr这个运算吗?
NO.PZ2018062016000083 0.216 0.784 C is correct. Baseon the corresponng formulp(x)=P(X=x)=(nx)px(1−p)n−xp{(x)}=P{(X=x)}={(\begin{array}{c}n\\x\enarray})}p^x{(1-p)}^{n-x}p(x)=P(X=x)=(nx)px(1−p)n−x, n = 3 anp = 0.30. p(1)=3!(3−1)!1!×0.31(1−0.3)2=(3)(0.3)(0.49)=0.441p(1)=\frac{3!}{(3-1)!1!}\times0.3^1(1-0.3)^2=\left(3\right)\left(0.3\right)\left(0.49\right)=0.441p(1)=(3−1)!1!3!×0.31(1−0.3)2=(3)(0.3)(0.49)=0.441 p(0)=3!(3−0)!0!×0.30(1−0.3)3=(1)(1)(0.343)=0.343⋅p(0)=\frac{3!}{(3-0)!0!}\times0.3^0(1-0.3)^3=\left(1\right)\left(1\right)\left(0.343\right)=0.343\ctp(0)=(3−0)!0!3!×0.30(1−0.3)3=(1)(1)(0.343)=0.343⋅ The requireprobability is: p(1) + p(0) = 0.441 + 0.343 = 0.784 问的是失败的概率那不应该是成功两次和成功三次的反面吗。那不就是失败一次和失败0次,而失败是0.7啊怎么不用0.7算。我选的B a
NO.PZ2018062016000083 这道题表达很奇怪啊 看不懂 说如果meet了上涨超过一次的goal,那么不meet goal的几率是多少 这表达完全错误啊 根本看不懂
NO.PZ2018062016000083 0.216 0.784 C is correct. Baseon the corresponng formulp(x)=P(X=x)=(nx)px(1−p)n−xp{(x)}=P{(X=x)}={(\begin{array}{c}n\\x\enarray})}p^x{(1-p)}^{n-x}p(x)=P(X=x)=(nx)px(1−p)n−x, n = 3 anp = 0.30. p(1)=3!(3−1)!1!×0.31(1−0.3)2=(3)(0.3)(0.49)=0.441p(1)=\frac{3!}{(3-1)!1!}\times0.3^1(1-0.3)^2=\left(3\right)\left(0.3\right)\left(0.49\right)=0.441p(1)=(3−1)!1!3!×0.31(1−0.3)2=(3)(0.3)(0.49)=0.441 p(0)=3!(3−0)!0!×0.30(1−0.3)3=(1)(1)(0.343)=0.343⋅p(0)=\frac{3!}{(3-0)!0!}\times0.3^0(1-0.3)^3=\left(1\right)\left(1\right)\left(0.343\right)=0.343\ctp(0)=(3−0)!0!3!×0.30(1−0.3)3=(1)(1)(0.343)=0.343⋅ The requireprobability is: p(1) + p(0) = 0.441 + 0.343 = 0.784 目标是大于等于1次,fail完成目标就应该是小于0次,就应该只计算p(0)的概率啊
NO.PZ2018062016000083 0.216 0.784 C is correct. Baseon the corresponng formulp(x)=P(X=x)=(nx)px(1−p)n−xp{(x)}=P{(X=x)}={(\begin{array}{c}n\\x\enarray})}p^x{(1-p)}^{n-x}p(x)=P(X=x)=(nx)px(1−p)n−x, n = 3 anp = 0.30. p(1)=3!(3−1)!1!×0.31(1−0.3)2=(3)(0.3)(0.49)=0.441p(1)=\frac{3!}{(3-1)!1!}\times0.3^1(1-0.3)^2=\left(3\right)\left(0.3\right)\left(0.49\right)=0.441p(1)=(3−1)!1!3!×0.31(1−0.3)2=(3)(0.3)(0.49)=0.441 p(0)=3!(3−0)!0!×0.30(1−0.3)3=(1)(1)(0.343)=0.343⋅p(0)=\frac{3!}{(3-0)!0!}\times0.3^0(1-0.3)^3=\left(1\right)\left(1\right)\left(0.343\right)=0.343\ctp(0)=(3−0)!0!3!×0.30(1−0.3)3=(1)(1)(0.343)=0.343⋅ The requireprobability is: p(1) + p(0) = 0.441 + 0.343 = 0.784请问二项分布公式(n x)px (1-p)n-x 这个(n x)什么意思呢