John Fraser’s performance is the first that Lazare and Warrack review. Fraser’s fund is constructed with a discretionary approach using the four Fama–French factors; he uses the Russell 1000 Value Index as his benchmark. The most recent 10 years of performance data for both the fund and the benchmark are shown in Exhibit 1.
Exhibit 1
Fraser Fund and Benchmark Average Monthly Performance over the Past 10 years
| Russell 1000 Value Index | The Fraser Fund | Factor Performance | |
| Monthly performance in excess of the risk-free rate | 0.72% | 0.55% | |
| β to specified factor | |||
| Market | 0.90 | 0.91 | 0.61% |
| Size | –0.27 | 0.15 | 0.17% |
| Value | 0.38 | 0.60 | 0.18% |
| Momentum | 0.15 | 0.08 | 0.72% |
| Average monthly risk-free rate | 0.33% | ||
As part of his evaluation of the applicants, Warrack compiles a record of the Active Share and active risk of the funds that they manage. He observes that the Mattley Fund has relatively high Active Share but relatively low active risk.
Q. Using Exhibit 1, the average monthly return of the Fraser Fund that is unexplained by rewarded factors is closest to:
- –0.20%.
- –0.17%.
- 0.13%.
Solution
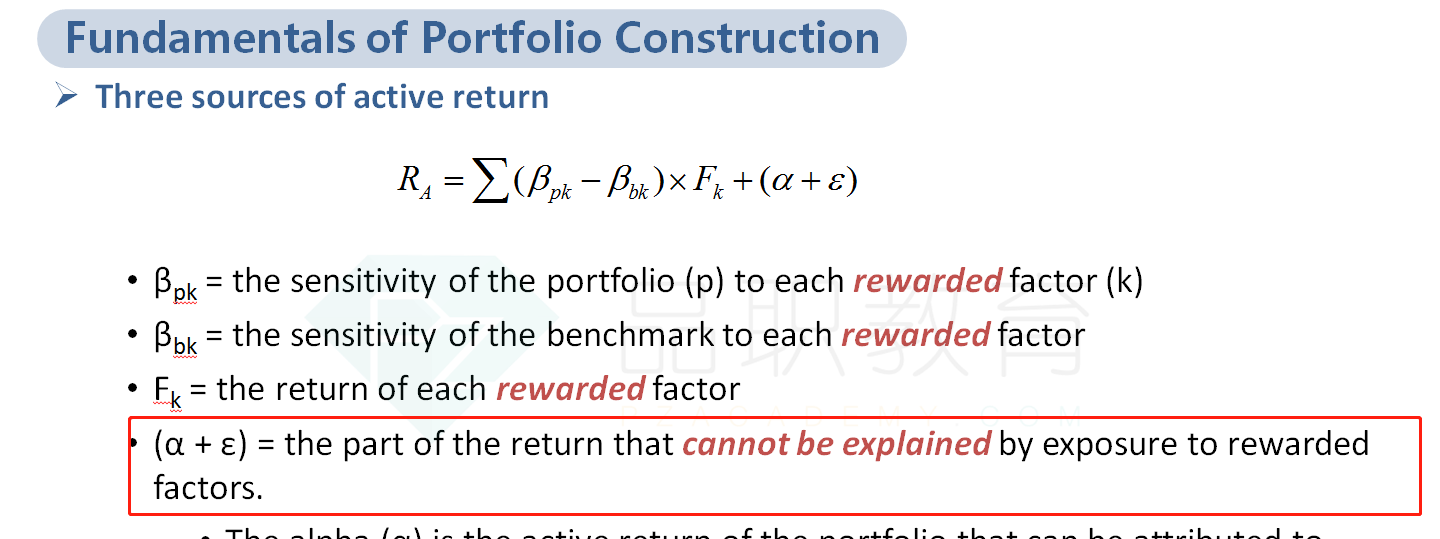
A is correct. Return from unrewarded factors = Actual monthly performance – Return from rewarded factors.
“Alpha” = RA – ∑βpkFk
where
RA = Actual portfolio performance
βpk = The sensitivity of the portfolio (p) to each rewarded factor (k)
Fk = The return for each rewarded factor
Return from rewarded factors = (0.91 × 0.61%) + (0.15 × 0.17%) + (0.60 × 0.18%) + (0.08 × 0.72%) = 0.75%.
“Alpha” = Return from unrewarded factors = 0.55% – 0.75% = –0.20%.
请问这题的解法是否因为Benchmark就是RF?