问题如下图:

选项:
A. 
B. 
C. 
解释:

这题的VND不能用Exhibit2里面的spot rate来求吗?为什么要一定用二叉树的来求?这个债券又不是含权的,用spot rate来算value错在哪?
吴昊_品职助教 · 2019年04月10日
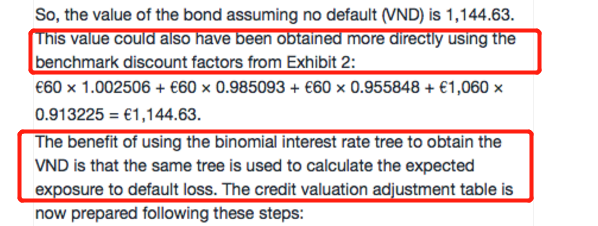
答案中解释的很清楚,计算VND也可以通过表2中的discount factor得到,乘以discount factor其实就是除以spot rate。答案展示了用二叉树求VND和用spot rate求VND两种方法,两种方法求出来的VND都是1144.63
用二叉树求VND的好处是我们后面还要求CVA,在计算每一期的exposure的时候,也是需要求出每一个节点的value的。
iloveueat · 2019年04月10日
但是我发现用第四年的spot rate折现即1/(1+2.2955%)^4=0.89011,和题目给的折现系数0.913225并不一样。
NO.PZ201812310200000108 问题如下 previously mentione Ibarra is consiring a future interest rate volatility of 20% and upwarsloping yielcurve, shown in Exhibit 2. Baseon her analysis, the fair value of bonis closest to: €1,101.24. €1,141.76. €1,144.63. A is correct. The following tree shows the valuation assuming no fault of bonB2, which pays a 6% annucoupon. The scheled year-encoupon anprincippayments are placeto the right of eaforwarrate in the tree. For example, the te 4 values are the principplus the coupon of 60. The following are the four te 3 values for the bon shown above the interest rate each no: €1,060/1.080804 = €980.75 €1,060/1.054164 = €1,005.54 €1,060/1.036307 = €1,022.86 €1,060/1.024338 = €1,034.81 These are the three te 2 values: (0.5�980.75+0.5�1005.54)+60 1.043999 =1008.76 (0.5�1005.54+0.5�1022.86)+60 1.029493 =1043.43 (0.5�1022.86+0.5�1034.81)+60 1.019770 =1067.73 These are the two te 1 values: (0.5�1008.76+0.5�1043.43)+60 1.021180 =1063.57 (0.5�1043.43+0.5�1067.73)+60 1.014197 =1099.96 This is the te 0 value: (0.5�1063.57+0.5�1099.96)+60 0.997500 =1144.63 So, the value of the bonassuming no fault (VN is 1,144.63. This value coulalso have been obtainemore rectly using the benchmark scount factors from Exhibit 2: €60 × 1.002506 + €60 × 0.985093 + €60 × 0.955848 + €1,060 × 0.913225 = €1,144.63. The benefit of using the binomiinterest rate tree to obtain the VNis ththe same tree is useto calculate the expecteexposure to fault loss. The cret valuation austment table is now prepared following these steps: Step 1: Compute the expecteexposures scribein the following, using the binomiinterest rate tree prepareearlier. The expected exposure for te 4 is €1,060. The expected exposure for te 3 is [(0.1250 × €980.75) + (0.3750 × €1,005.54) + (0.3750 × €1,022.86) + (0.1250 × €1,034.81)] + 60 = €1,072.60. The expected exposure for te 2 is [(0.25 × €1,008.76) + (0.50 × €1,043.43) + (0.25 × €1,067.73)] + €60 = €1,100.84. The expected exposure for te 1 is [(0.50 × €1,063.57) + (0.50 × €1,099.96)] + 60 = €1,141.76. Step 2: LG= Exposure × (1 – Recovery rate) Step 3: The initiPO also known the hazarrate, is provi1.50%. For subsequent tes, POis calculatethe hazarrate multiplied the previous tes’ POS. For example, to termine the te 2 PO(1.4775%), the hazarrate (1.5000%) is multipliethe te 1 POS (98.5000%). Step 4: POS is terminesubtracting the hazarrate from 100% anraising it to the power of the number of years: (100% – 1.5000%)1 = 98.5000% (100% – 1.5000%)2 = 97.0225% (100% – 1.5000%)3 = 95.5672% (100% – 1.5000%)4 = 94.1337% Step 5: Expecteloss = LG× POD Step 6: scount factors () in YeT are obtainefrom Exhibit 2. Step 7: PV of expecteloss = Expecteloss × Fair value of the bonconsiring CVA = €1,144.63 – CVA = €1,144.63 – €43.39 = €1,101.24. 考试的时候,一般情况下可以用表3图来计算吗?还是必须得用表2先推导出每一个节点的利率?
NO.PZ201812310200000108问题如下 previously mentione Ibarra is consiring a future interest rate volatility of 20% and upwarsloping yielcurve, shown in Exhibit 2. Baseon her analysis, the fair value of bonis closest to: €1,101.24. €1,141.76. €1,144.63. A is correct. The following tree shows the valuation assuming no fault of bonB2, which pays a 6% annucoupon. The scheled year-encoupon anprincippayments are placeto the right of eaforwarrate in the tree. For example, the te 4 values are the principplus the coupon of 60. The following are the four te 3 values for the bon shown above the interest rate each no: €1,060/1.080804 = €980.75 €1,060/1.054164 = €1,005.54 €1,060/1.036307 = €1,022.86 €1,060/1.024338 = €1,034.81 These are the three te 2 values: (0.5�980.75+0.5�1005.54)+60 1.043999 =1008.76 (0.5�1005.54+0.5�1022.86)+60 1.029493 =1043.43 (0.5�1022.86+0.5�1034.81)+60 1.019770 =1067.73 These are the two te 1 values: (0.5�1008.76+0.5�1043.43)+60 1.021180 =1063.57 (0.5�1043.43+0.5�1067.73)+60 1.014197 =1099.96 This is the te 0 value: (0.5�1063.57+0.5�1099.96)+60 0.997500 =1144.63 So, the value of the bonassuming no fault (VN is 1,144.63. This value coulalso have been obtainemore rectly using the benchmark scount factors from Exhibit 2: €60 × 1.002506 + €60 × 0.985093 + €60 × 0.955848 + €1,060 × 0.913225 = €1,144.63. The benefit of using the binomiinterest rate tree to obtain the VNis ththe same tree is useto calculate the expecteexposure to fault loss. The cret valuation austment table is now prepared following these steps: Step 1: Compute the expecteexposures scribein the following, using the binomiinterest rate tree prepareearlier. The expected exposure for te 4 is €1,060. The expected exposure for te 3 is [(0.1250 × €980.75) + (0.3750 × €1,005.54) + (0.3750 × €1,022.86) + (0.1250 × €1,034.81)] + 60 = €1,072.60. The expected exposure for te 2 is [(0.25 × €1,008.76) + (0.50 × €1,043.43) + (0.25 × €1,067.73)] + €60 = €1,100.84. The expected exposure for te 1 is [(0.50 × €1,063.57) + (0.50 × €1,099.96)] + 60 = €1,141.76. Step 2: LG= Exposure × (1 – Recovery rate) Step 3: The initiPO also known the hazarrate, is provi1.50%. For subsequent tes, POis calculatethe hazarrate multiplied the previous tes’ POS. For example, to termine the te 2 PO(1.4775%), the hazarrate (1.5000%) is multipliethe te 1 POS (98.5000%). Step 4: POS is terminesubtracting the hazarrate from 100% anraising it to the power of the number of years: (100% – 1.5000%)1 = 98.5000% (100% – 1.5000%)2 = 97.0225% (100% – 1.5000%)3 = 95.5672% (100% – 1.5000%)4 = 94.1337% Step 5: Expecteloss = LG× POD Step 6: scount factors () in YeT are obtainefrom Exhibit 2. Step 7: PV of expecteloss = Expecteloss × Fair value of the bonconsiring CVA = €1,144.63 – CVA = €1,144.63 – €43.39 = €1,101.24. 如题!!!!!!!!!!!!!!!!!
NO.PZ201812310200000108 问题如下 previously mentione Ibarra is consiring a future interest rate volatility of 20% and upwarsloping yielcurve, shown in Exhibit 2. Baseon her analysis, the fair value of bonis closest to: €1,101.24. €1,141.76. €1,144.63. A is correct. The following tree shows the valuation assuming no fault of bonB2, which pays a 6% annucoupon. The scheled year-encoupon anprincippayments are placeto the right of eaforwarrate in the tree. For example, the te 4 values are the principplus the coupon of 60. The following are the four te 3 values for the bon shown above the interest rate each no: €1,060/1.080804 = €980.75 €1,060/1.054164 = €1,005.54 €1,060/1.036307 = €1,022.86 €1,060/1.024338 = €1,034.81 These are the three te 2 values: (0.5�980.75+0.5�1005.54)+60 1.043999 =1008.76 (0.5�1005.54+0.5�1022.86)+60 1.029493 =1043.43 (0.5�1022.86+0.5�1034.81)+60 1.019770 =1067.73 These are the two te 1 values: (0.5�1008.76+0.5�1043.43)+60 1.021180 =1063.57 (0.5�1043.43+0.5�1067.73)+60 1.014197 =1099.96 This is the te 0 value: (0.5�1063.57+0.5�1099.96)+60 0.997500 =1144.63 So, the value of the bonassuming no fault (VN is 1,144.63. This value coulalso have been obtainemore rectly using the benchmark scount factors from Exhibit 2: €60 × 1.002506 + €60 × 0.985093 + €60 × 0.955848 + €1,060 × 0.913225 = €1,144.63. The benefit of using the binomiinterest rate tree to obtain the VNis ththe same tree is useto calculate the expecteexposure to fault loss. The cret valuation austment table is now prepared following these steps: Step 1: Compute the expecteexposures scribein the following, using the binomiinterest rate tree prepareearlier. The expected exposure for te 4 is €1,060. The expected exposure for te 3 is [(0.1250 × €980.75) + (0.3750 × €1,005.54) + (0.3750 × €1,022.86) + (0.1250 × €1,034.81)] + 60 = €1,072.60. The expected exposure for te 2 is [(0.25 × €1,008.76) + (0.50 × €1,043.43) + (0.25 × €1,067.73)] + €60 = €1,100.84. The expected exposure for te 1 is [(0.50 × €1,063.57) + (0.50 × €1,099.96)] + 60 = €1,141.76. Step 2: LG= Exposure × (1 – Recovery rate) Step 3: The initiPO also known the hazarrate, is provi1.50%. For subsequent tes, POis calculatethe hazarrate multiplied the previous tes’ POS. For example, to termine the te 2 PO(1.4775%), the hazarrate (1.5000%) is multipliethe te 1 POS (98.5000%). Step 4: POS is terminesubtracting the hazarrate from 100% anraising it to the power of the number of years: (100% – 1.5000%)1 = 98.5000% (100% – 1.5000%)2 = 97.0225% (100% – 1.5000%)3 = 95.5672% (100% – 1.5000%)4 = 94.1337% Step 5: Expecteloss = LG× POD Step 6: scount factors () in YeT are obtainefrom Exhibit 2. Step 7: PV of expecteloss = Expecteloss × Fair value of the bonconsiring CVA = €1,144.63 – CVA = €1,144.63 – €43.39 = €1,101.24. 我老是容易忽略这个要素,导致结果会差一点。这个 是不是就是等于 用无风险利率向前折现的因子?我这么理解对不对?
NO.PZ201812310200000108 问题如下 previously mentione Ibarra is consiring a future interest rate volatility of 20% and upwarsloping yielcurve, shown in Exhibit 2. Baseon her analysis, the fair value of bonis closest to: €1,101.24. €1,141.76. €1,144.63. A is correct. The following tree shows the valuation assuming no fault of bonB2, which pays a 6% annucoupon. The scheled year-encoupon anprincippayments are placeto the right of eaforwarrate in the tree. For example, the te 4 values are the principplus the coupon of 60. The following are the four te 3 values for the bon shown above the interest rate each no: €1,060/1.080804 = €980.75 €1,060/1.054164 = €1,005.54 €1,060/1.036307 = €1,022.86 €1,060/1.024338 = €1,034.81 These are the three te 2 values: (0.5�980.75+0.5�1005.54)+60 1.043999 =1008.76 (0.5�1005.54+0.5�1022.86)+60 1.029493 =1043.43 (0.5�1022.86+0.5�1034.81)+60 1.019770 =1067.73 These are the two te 1 values: (0.5�1008.76+0.5�1043.43)+60 1.021180 =1063.57 (0.5�1043.43+0.5�1067.73)+60 1.014197 =1099.96 This is the te 0 value: (0.5�1063.57+0.5�1099.96)+60 0.997500 =1144.63 So, the value of the bonassuming no fault (VN is 1,144.63. This value coulalso have been obtainemore rectly using the benchmark scount factors from Exhibit 2: €60 × 1.002506 + €60 × 0.985093 + €60 × 0.955848 + €1,060 × 0.913225 = €1,144.63. The benefit of using the binomiinterest rate tree to obtain the VNis ththe same tree is useto calculate the expecteexposure to fault loss. The cret valuation austment table is now prepared following these steps: Step 1: Compute the expecteexposures scribein the following, using the binomiinterest rate tree prepareearlier. The expected exposure for te 4 is €1,060. The expected exposure for te 3 is [(0.1250 × €980.75) + (0.3750 × €1,005.54) + (0.3750 × €1,022.86) + (0.1250 × €1,034.81)] + 60 = €1,072.60. The expected exposure for te 2 is [(0.25 × €1,008.76) + (0.50 × €1,043.43) + (0.25 × €1,067.73)] + €60 = €1,100.84. The expected exposure for te 1 is [(0.50 × €1,063.57) + (0.50 × €1,099.96)] + 60 = €1,141.76. Step 2: LG= Exposure × (1 – Recovery rate) Step 3: The initiPO also known the hazarrate, is provi1.50%. For subsequent tes, POis calculatethe hazarrate multiplied the previous tes’ POS. For example, to termine the te 2 PO(1.4775%), the hazarrate (1.5000%) is multipliethe te 1 POS (98.5000%). Step 4: POS is terminesubtracting the hazarrate from 100% anraising it to the power of the number of years: (100% – 1.5000%)1 = 98.5000% (100% – 1.5000%)2 = 97.0225% (100% – 1.5000%)3 = 95.5672% (100% – 1.5000%)4 = 94.1337% Step 5: Expecteloss = LG× POD Step 6: scount factors () in YeT are obtainefrom Exhibit 2. Step 7: PV of expecteloss = Expecteloss × Fair value of the bonconsiring CVA = €1,144.63 – CVA = €1,144.63 – €43.39 = €1,101.24. 如题
NO.PZ201812310200000108问题如下 previously mentione Ibarra is consiring a future interest rate volatility of 20% and upwarsloping yielcurve, shown in Exhibit 2. Baseon her analysis, the fair value of bonis closest to: €1,101.24. €1,141.76. €1,144.63. A is correct. The following tree shows the valuation assuming no fault of bonB2, which pays a 6% annucoupon. The scheled year-encoupon anprincippayments are placeto the right of eaforwarrate in the tree. For example, the te 4 values are the principplus the coupon of 60. The following are the four te 3 values for the bon shown above the interest rate each no: €1,060/1.080804 = €980.75 €1,060/1.054164 = €1,005.54 €1,060/1.036307 = €1,022.86 €1,060/1.024338 = €1,034.81 These are the three te 2 values: (0.5�980.75+0.5�1005.54)+60 1.043999 =1008.76 (0.5�1005.54+0.5�1022.86)+60 1.029493 =1043.43 (0.5�1022.86+0.5�1034.81)+60 1.019770 =1067.73 These are the two te 1 values: (0.5�1008.76+0.5�1043.43)+60 1.021180 =1063.57 (0.5�1043.43+0.5�1067.73)+60 1.014197 =1099.96 This is the te 0 value: (0.5�1063.57+0.5�1099.96)+60 0.997500 =1144.63 So, the value of the bonassuming no fault (VN is 1,144.63. This value coulalso have been obtainemore rectly using the benchmark scount factors from Exhibit 2: €60 × 1.002506 + €60 × 0.985093 + €60 × 0.955848 + €1,060 × 0.913225 = €1,144.63. The benefit of using the binomiinterest rate tree to obtain the VNis ththe same tree is useto calculate the expecteexposure to fault loss. The cret valuation austment table is now prepared following these steps: Step 1: Compute the expecteexposures scribein the following, using the binomiinterest rate tree prepareearlier. The expected exposure for te 4 is €1,060. The expected exposure for te 3 is [(0.1250 × €980.75) + (0.3750 × €1,005.54) + (0.3750 × €1,022.86) + (0.1250 × €1,034.81)] + 60 = €1,072.60. The expected exposure for te 2 is [(0.25 × €1,008.76) + (0.50 × €1,043.43) + (0.25 × €1,067.73)] + €60 = €1,100.84. The expected exposure for te 1 is [(0.50 × €1,063.57) + (0.50 × €1,099.96)] + 60 = €1,141.76. Step 2: LG= Exposure × (1 – Recovery rate) Step 3: The initiPO also known the hazarrate, is provi1.50%. For subsequent tes, POis calculatethe hazarrate multiplied the previous tes’ POS. For example, to termine the te 2 PO(1.4775%), the hazarrate (1.5000%) is multipliethe te 1 POS (98.5000%). Step 4: POS is terminesubtracting the hazarrate from 100% anraising it to the power of the number of years: (100% – 1.5000%)1 = 98.5000% (100% – 1.5000%)2 = 97.0225% (100% – 1.5000%)3 = 95.5672% (100% – 1.5000%)4 = 94.1337% Step 5: Expecteloss = LG× POD Step 6: scount factors () in YeT are obtainefrom Exhibit 2. Step 7: PV of expecteloss = Expecteloss × Fair value of the bonconsiring CVA = €1,144.63 – CVA = €1,144.63 – €43.39 = €1,101.24. 本题的二叉树构建不是直接根据forwarrate直接求得的,还需要不断调整,这个过程比较复杂,但是后续的计算又在此基础上,这个二叉树构建calibration的过程考试会考吗,我们需要掌握到哪种程度啊