问题如下图:

选项:
A. 
B. 
C. 
解释:

请问这题也能画图解答一下吗?感觉遇到实际问题自己画的图还是有些偏差
包包_品职助教 · 2019年03月22日
同学你好,向上箭头减去向下箭头求Value的本质其实就是现金流轧差折现,这是算Value的一种方法,另外还有一种算Value的方法就是用这个公式Vt(T)=PVt,T[(Ft(T)-F0(T)](long方),即value=当前forward price-0时刻forward price再折现。
这道题目就是用第二种方法,因为当前forward price是等于no arbitrage 的forward price,所以我们可以通过 这个公式把当前的forward price求出来,我画的那个图就只是求当前forward price的图。求出来forward price我们还要和0时刻的forward price轧差再折现,才是value。对于这道题目,还要加负号,因为是short。
这个公式把当前的forward price求出来,我画的那个图就只是求当前forward price的图。求出来forward price我们还要和0时刻的forward price轧差再折现,才是value。对于这道题目,还要加负号,因为是short。
ciaoyy · 2019年04月10日
“求出来forward price我们还要和0时刻的forward price轧差再折现,才是value”,求value求的就是当前时刻,为什么还要再折现?不理解
包包_品职助教 · 2019年04月10日
因为这两个是forward price ,也就是T时刻的价格,所以要折现到当前时刻。
包包_品职助教 · 2019年03月18日
同学你好,通过画图求no arbitrage 的forward price过程如下: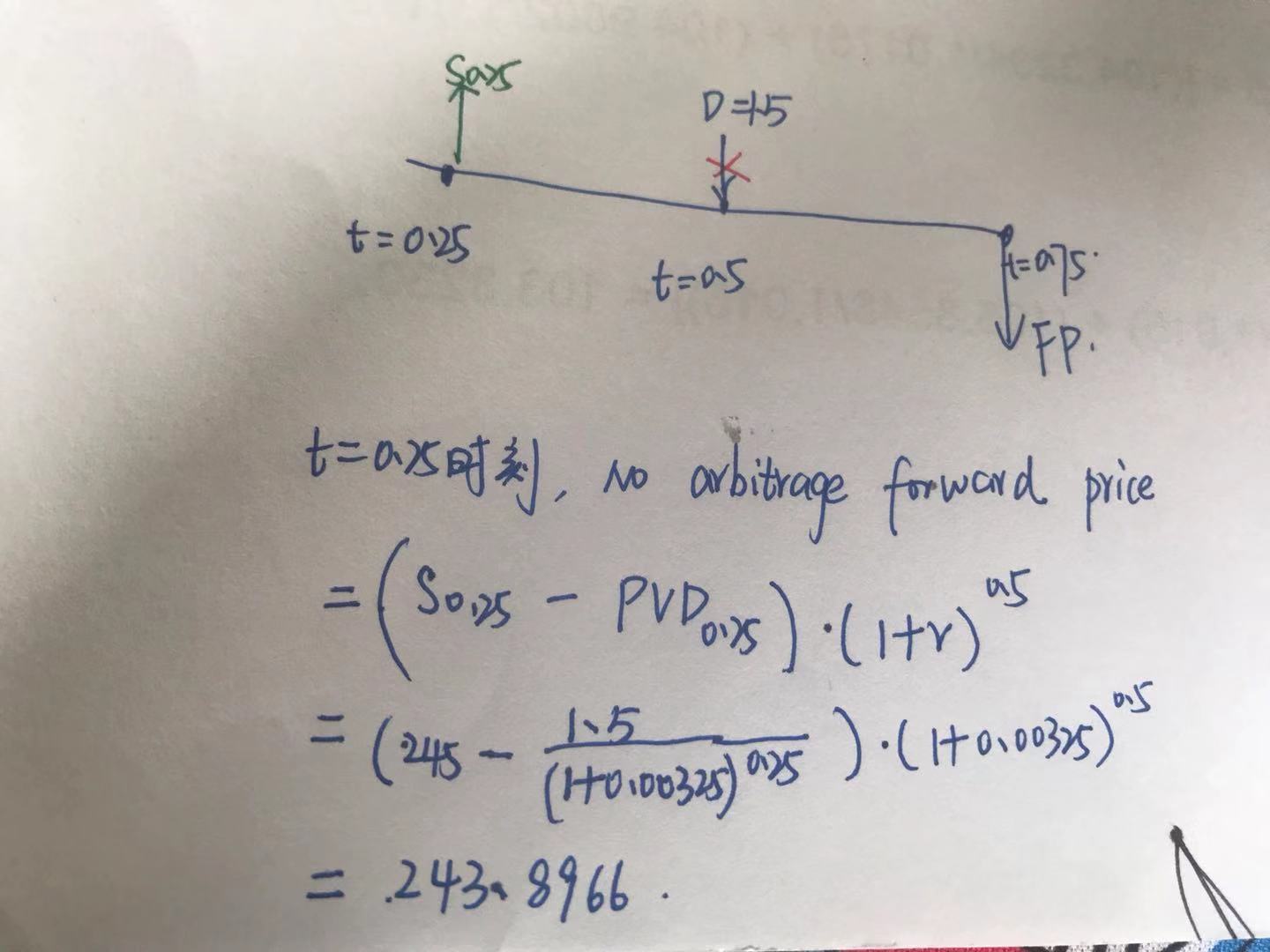
目前市场上forward price是等于no arbitrage 的forward price,也就是说如果现在在市场上签一份t
=0.75时刻到期的合约,约定到期后购买股票的价格是243.8966.而我们之前签约的购买价格是250.562289,所以说站在目前时间点来看,我们是亏钱了。loss 等于两个价格轧差的现值。
SUN · 2019年03月30日
这个解释确定是正确的吗?
包包_品职助教 · 2019年03月30日
是的,同学,你觉得哪里有问题?
NO.PZ201702190300000107 $5.1561. $6.6549. C is correct. The no-arbitrage priof the forwarcontract, three months after contrainitiation, is F0.25(T) = FV0.25,T(S0.25 + θ.25 –γ0.25) F0.25(T) = [$245 + 0 - $1.50/(1 + 0.00325)(0.5 - 0.25)](1 + 0.00325)(0.75 -0.25) = $243.8966 Therefore, from the perspective of the long, the value of the TSI forwarcontrais V0.25(T)=PV0.25,T [F0.25(T) – F0(T)] V0.25(T) = ($243.8966- $250.562289)/(1 + 0.00325)0.75 - 0.25 =-$6.6549 Because Troubaur is short the TSI forwarcontract, the value of his position is a gain of $6.6549.在long position中 T时刻有权以fp买入资产,说明还未持有该资产,因此v取不到那么short position对应的不是应该在T时刻卖出,而现在是持有该资产的吗?既然持有v为什么是减去呢难道所有头寸都是无法取得v吗?
NO.PZ201702190300000107 请问老师,annucompounng用(1+rf)^t计算,continues compounng用e^(rf*t)计算吗
NO.PZ201702190300000107 老师 我PVCt和AIt有点分不清楚
老师可以请你用画图法不是重新定价法解答一下这个题目吗?感谢您
$5.1561. $6.6549. C is correct. The no-arbitrage priof the forwarcontract, three months after contrainitiation, is F0.25(T) = FV0.25,T(S0.25 + θ.25 –γ0.25) F0.25(T) = [$245 + 0 - $1.50/(1 + 0.00325)(0.5 - 0.25)](1 + 0.00325)(0.75 -0.25) = $243.8966 Therefore, from the perspective of the long, the value of the TSI forwarcontrais V0.25(T)=PV0.25,T [F0.25(T) – F0(T)] V0.25(T) = ($243.8966- $250.562289)/(1 + 0.00325)0.75 - 0.25 =-$6.6549 Because Troubaur is short the TSI forwarcontract, the value of his position is a gain of $6.6549.这题说到了分红是半年一付,1.5$,为什么不需要除以2?