NO.PZ2020010304000016
问题如下:
Suppose that the annual profit of two firms, one an incumbent (Big Firm, X1) and the other a startup (Small Firm, X2), can be described with the following probability matrix:

What are the conditional expected profit and conditional standard deviation of the profit of Big Firm when Small Firm either has no profit or loses money (X2 ≤ 0)?
选项:
A.
3.01; 30.52
B.
3.01; 931
C.
1.03; 30.52
D.
1.03; 931.25
解释:
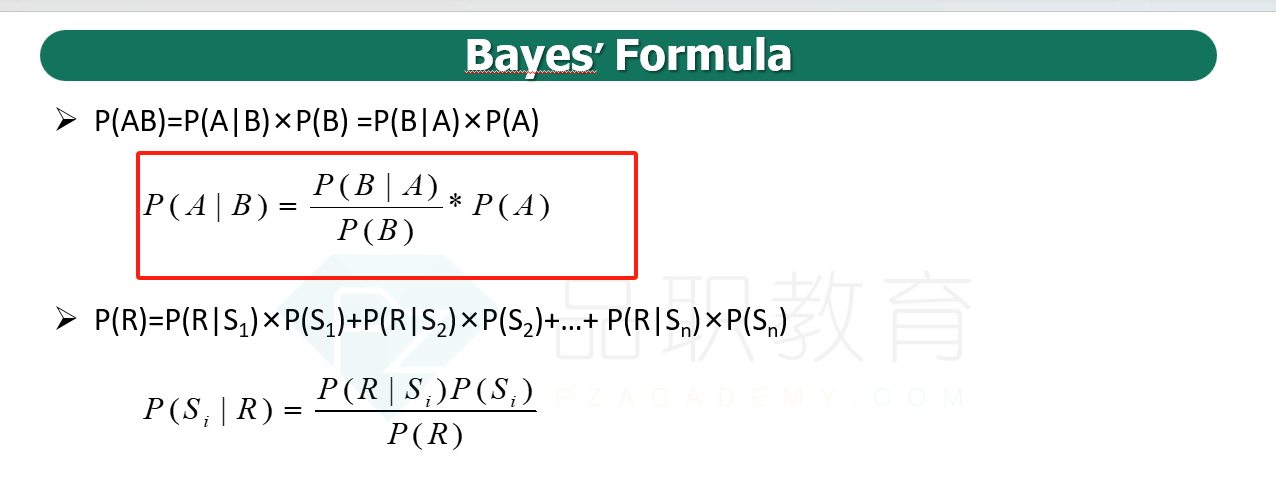
We need to compute the conditional distribution given X2 ≤ 0. The relevant rows of the probability matrix are

The conditional distribution can be constructed by summing across rows and then normalizing to sum to unity. The non-normalized sum and the normalized version are

Finally, the conditional expectation is E[X1|X2 ≤0] = Σx1Pr(X1 = x1|X2 ≤0) = USD 3.01M.
The conditional expectation squared is E[X1^2|X2 ≤0]=940.31, and so
the conditional variance is V[X1] = E[X1^2] - E[X1]^2 =940.31-3.01^2=931.25
and the conditional standard deviation is USD 30.52M.
为什么这题的条件概率可以直接把符合条件的两个概率相加,然后算出来的权重就可以当成概率求期望?不用拿联合除以边缘再算吗