题目和选项都不太理解

问题如下：

Andrew Omandi Case Scenario

Andrew Omandi works as a senior analyst at investment firm CPSR Partners. He and junior analysts, Emmanuel Katangole, Takasongo Kasongo and Peter Mensah, have been tasked with investigating the use of multi factor models to help explain portfolio returns. After conducting some research, they identify a three-factor model described below, and are meeting to finalize their presentation to the firm's investment committee.

R_it - R_ft = intercept_it + B_M(R_Mt-R_ft) + B_SMB(SMB_t) + B_HML(HML_t) + e_it

Where:

R_it = portfolio return

R_ft = risk free rate, one-month T-bill return

B_M = market regression coefficient

R_Mt = return on market portfolio

R_Mt-R_ft = market risk premium

B_SMB = SMB regression coefficient

SMB_t = return difference between small cap stocks and large cap stocks (size premium)

B_HML = HML regression coefficient

HML_t = return difference between high book to market stocks and low book to market stocks (value premium)

e_it = error term

Omandi states: "This model indicates that the main factors driving expected portfolio excess returns are premiums for market risk (R_Mt-R_ft), size (SMB_t) and value (HML_t). I also believe that there is a positive relationship between portfolio excess return and each of the independent variables, market risk, size and value premiums. Based on this we can formulate the following hypotheses:

Hypothesis 1

H_o: B_M = 0

H_a: B_M ≠ 0

Hypothesis 2

H_o: B_{SMB ≤} 0

H_a: B_SMB > 0

Hypothesis 3

H_o: B_{HML >} 0

H_a: B_{HML ≤} 0"

The analysts test the model and the regression results of excess portfolio returns on Mkt-Rf, SMB and HML are presented below in Exhibit 1.

Exhibit 1:

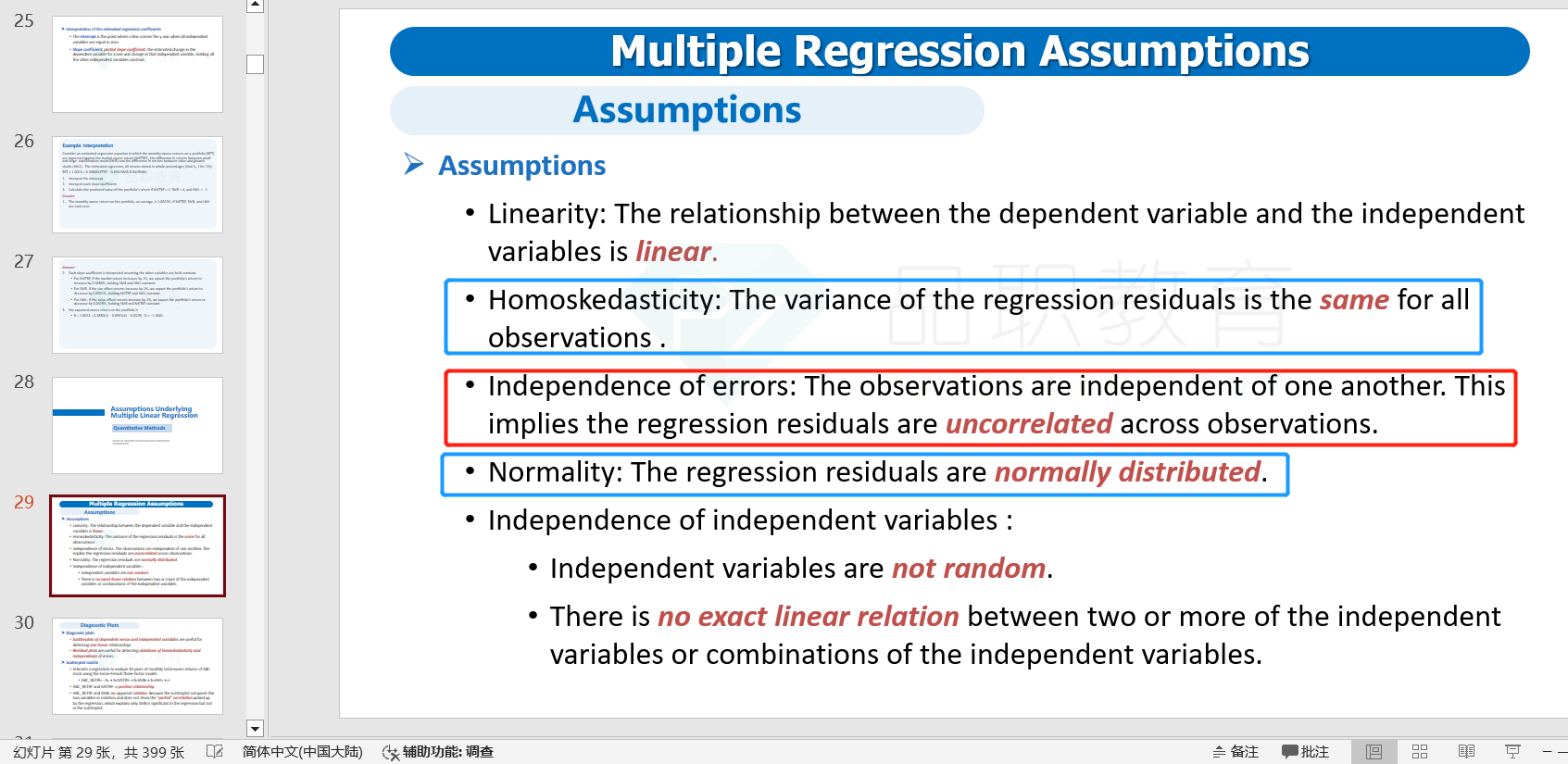
Kasango states that it is important to emphasize that the multiple linear regression model makes a number of assumptions, three of which are:

• Assumption 1: The regression residuals are normally distributed

• Assumption 2: The variance of the regression residuals is the same for all observations.

• Assumption 3: The regression residuals are correlated across observations.

Katangole asks how one can assess the goodness of fit of the estimated regression to the data. Mensah responds, "One measure, the R² can be defined as the ratio of the variation in the dependent variable explained by the independent variables to the total variation of the dependent variable. However, e R² stays the same or increases when independent variables are added to the regression. A better measure is the adjusted R² which does not automatically increase when independent variables are added to the regression."

Kasango is least likely correct with regard to which assumption?

选项：

A.A.Assumption 1

B.B.Assumption 2

C.C.Assumption 3

解释：

• A is Incorrect because one of the assumptions of the multiple regression model is the the regression residuals are error term e_it is normally distributed. That is assumption 1 is correct.

• B is Incorrect because another of the multiple regression model is that the variance of the regression residuals error term e_it is the same for all observations. That is assumption 2 is correct.

• C is Correct, as there are six assumptions of the multiple linear regression model, this question addresses three of them. Two of the assumptions are stated correctly:

  • Assumption 1: The regression residuals are error term is normally distributed
  • Assumption 2: The variance of the residuals error term is the same for all observations.

  The third one, assumption 3 is incorrect. Correctly stated it should read: The regression residuals are uncorrelated across observations.: the expected value of the error term, conditioned on the independent variables, is equal to zero.