NO.PZ2024082801000022
问题如下:
Question An analyst estimates an AR(2) model for the monthly sales of a company and calculates the squared residuals from this regression. To test for the presence of ARCH(1) in the residuals, the analyst should regress the squared residuals on a constant and:
选项:
A.A.a time trend, and test whether the time trend coefficient is statistically different from 0.
B.B.one lag of the squared residuals, and test whether the slope coefficient is statistically different from 0.
C.C.one lag of the squared residuals, and test whether the slope coefficient is statistically different from 1.
解释:
A is Incorrect because, to test for ARCH(1), we do the following: Regress the squared residual from your time-series model on a lagged value of the squared residual. Test whether the coefficient on the squared lagged residual differs significantly from 0. So, the independent variable is one lag of the squared residuals, not a time trend.
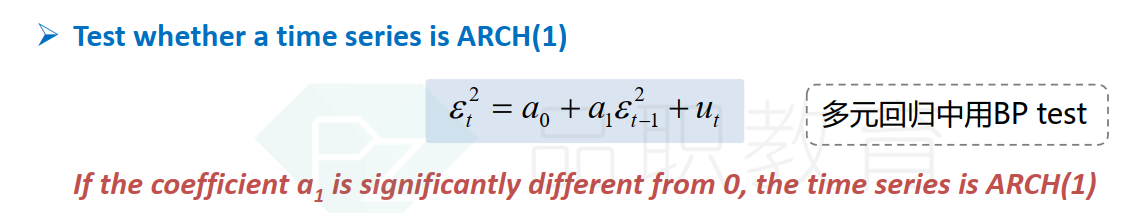
B is Correct because, to test for ARCH(1), we do the following: Regress the squared residual from your time-series model on a lagged value of the squared residual and test whether the coefficient on the squared lagged residual differs significantly from 0.
C is Incorrect because, to test for ARCH(1), we do the following: Regress the squared residual from your time-series model on a lagged value of the squared residual. Test whether the coefficient on the squared lagged residual differs significantly from 0. So, the time series has an ARCH(1) process if the slope coefficient is statistically significantly different from 0, not 1.
具体的知识点是哪一个视频