NO.PZ2023091601000122
问题如下:
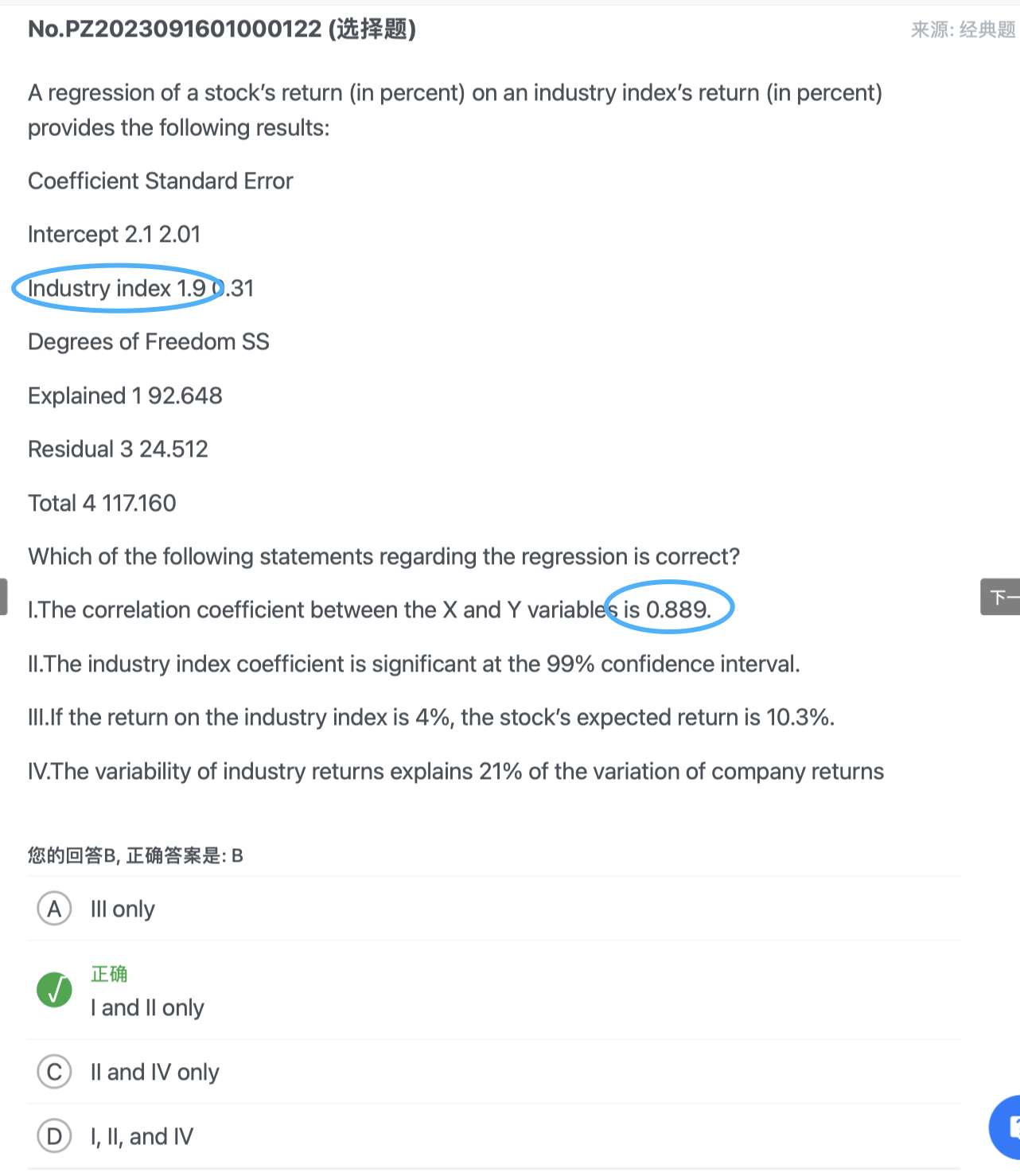
A regression of a stock’s
return (in percent) on an industry index’s return (in percent) provides the
following results:
Coefficient Standard Error
Intercept 2.1 2.01
Industry index 1.9 0.31
Degrees of Freedom SS
Explained 1 92.648
Residual
3 24.512
Total 4 117.160
Which of the
following statements regarding the regression is correct?
I.The correlation
coefficient between the X and Y variables is 0.889.
II.The industry index
coefficient is significant at the 99% confidence interval.
III.If the return on
the industry index is 4%, the stock’s expected return is 10.3%.
IV.The
variability of industry returns explains 21% of the variation of company
returns
选项:
A.III
only
I and II only
II and IV only
I, II, and IV
解释:
The R2 of the regression
is calculated as ESS/TSS = (92.648/117.160) = 0.79, which means that the
variation in industry returns explains 79% of the variation in the stock
return. By taking the square root of R2, we can calculate that the
correlation coefficient (r) = 0.889. The t-statistic for the industry return
coefficient is 1.91/0.31 = 6.13, which is sufficiently large enough for the
coefficient to be significant at the 99% confidence interval. Since we have the
regression coefficient and intercept, we know that the regression equation is Rstock = l.9X + 2.1.
Plugging in a value of 4% for the industry return, we get a stock return of 1.9
(4) + 2.1 = 9.7%.
请问老师,rho是R方的开方,在一元中和b1是不是应该一样啊?



