NO.PZ2023100703000022
问题如下:
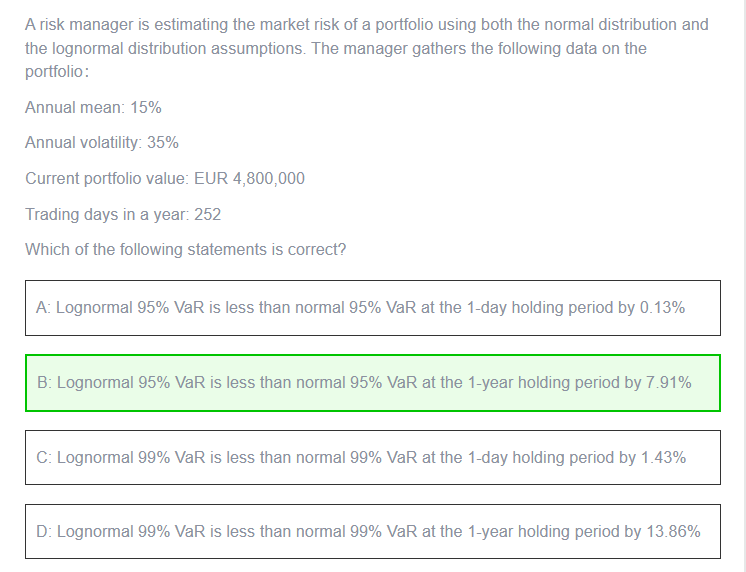
A risk manager is estimating the market risk of a portfolio using both the normal distribution and the lognormal distribution assumptions. The manager gathers the following data on the portfolio: Annual mean: 15% Annual volatility: 35% Current portfolio value: EUR 4,800,000 Trading days in a year: 252 Which of the following statements is correct?选项:
A.Lognormal 95% VaR is less than normal 95% VaR at the 1-day holding period by 0.13% B.Lognormal 95% VaR is less than normal 95% VaR at the 1-year holding period by 7.91% C.Lognormal 99% VaR is less than normal 99% VaR at the 1-day holding period by 1.43% D.Lognormal 99% VaR is less than normal 99% VaR at the 1-year holding period by 13.86%解释:
Annual return = 0.15; Daily
return = 0.15/252 = 0.000595
Annual volatility = 0.35;
Daily volatility = 0.35/sqrt(252) = 0.022048
The normal VaR and
lognormal VaR, in percentage terms, are calculated as follows (ignoring negative
signs):
1-day normal 95% VaR = (Rp
– zs) = (0.000595 – 1.645 * 0.022048) = 3.57%
1-day lognormal 95% VaR =
(1 – e[Rp – zs]) = (1 – exp[0.000595 – 1.645*0.022048])m = 3.51%
1-year normal 95% VaR = (Rp
– zs) = (0.15 – 1.645 * 0.35) = 42.58%
1-year lognormal 95% VaR =
(1 – exp[0.15 – (1.645*0.35)]) = 34.67%
Also,
1-day normal 99% VaR = (Rp
– zs) = (0.000595 – 2.326 * 0.022048) = 5.07%
1-day lognormal 99% VaR =
(1 – e[Rp – zs]) = (1 – exp[0.000595 – 2.326*0.022048]) = 4.94%
1-year normal 99% VaR = (Rp
– zs) = (0.15 – 2.326 * 0.35) = 66.41%
1-year lognormal 99% VaR =
(1 – exp[0.15 – (2.326*0.35)]) = 48.53%
Hence,
1-day holding period:
Lognormal 95% VaR is smaller than Normal 95% VaR by: 3.57 – 3.51 = 0.06%. So, A
is incorrect. (See explanation for C below).
1-year holding period:
Lognormal 95% VaR is smaller than Normal 95% VaR by: 42.58 – 34.67 = 7.91%. So,
B is correct.
1-day holding period:
Lognormal 99% VaR is smaller than Normal 99% VaR by: 5.07 – 4.94 = 0.13%. So, C
is incorrect (4.94 – 3.51 = 1.43%).
1-year holding period:
Lognormal 99% VaR is smaller than Normal 99% VaR by: 66.41 – 48.53 = 17.88%. So,
D is incorrect (48.53 – 34.67 = 13.86%).
The normal VaR and
lognormal VaR, in value terms, are calculated as follows (ignoring negative
signs):
1-day normal 95% VaR = (Rp
– zs)*V = (0.000595 – 1.645 * 0.022048)*4.8m = EUR 171,235
1-day lognormal 95% VaR =
(1 – e[Rp – zs])*V = (1 – exp[0.000595 – 1.645*0.022048])*4.8m = EUR
168,217
1-year normal 95% VaR = (Rp
– zs)*V = (0.15 – 1.645 * 0.35)*4.8m = EUR 2,043,600
1-year lognormal 95% VaR =
(1 – exp[0.15 – (1.645*0.35)])*4.8m = EUR 1,664,258
Also,1-day normal 99% VaR = (Rp – zs)*V = (0.000595 – 2.326 *
0.022048)*4.8m = EUR 243,306
1-day lognormal 99% VaR =
(1 – e[Rp – zs])*V = (1 – exp[0.000595 – 2.326*0.022048])*4.8m = EUR
237,242
1-year normal 99% VaR = (Rp
– zs)*V = (0.15 – 2.326 * 0.35)*4.8m = EUR 3,187,680
1-year lognormal 99% VaR =
(1 – exp[0.15 – (2.326*0.35)])*4.8m = EUR 2,329,264
Hence,1-day holding period: Lognormal 95% VaR is smaller than Normal 95% VaR
by: 171,235 – 168,217 = EUR 3,018.
1-year holding period: Lognormal
95% VaR is smaller than Normal 95% VaR by: 2,043,600 – 1,664,258 = EUR 379,342.
1-day holding period:
Lognormal 99% VaR is smaller than Normal 99% VaR by: 243,306 – 237,242 = EUR
6,064.
1-year holding period:
Lognormal 99% VaR is smaller than Normal 99% VaR by: 3,187,680 – 2,329,264 =
EUR 858,416.
题错了吧