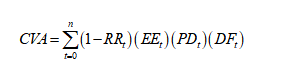NO.PZ2024050101000100
问题如下:
A CRO at an investment bank has asked the risk department to evaluate the bank’s 3-year derivative exposure position with a counterparty. The 1-year CDS on the counterparty is currently trading at a spread of 180 bps. The table below presents trade and forecast data on the CDS spread, the expected exposure, and the recovery rate on the counterparty:

Additionally, the CRO has presented the risk team with the following set of assumptions to use in conducting the analysis:
• Counterparty’s default probabilities follow a constant hazard rate process
• The investment bank and the counterparty have signed a credit support annex (CSA) to cover this exposure, which requires collateral posting of AUD 13 million over the life of the contract
• The current risk-free rate of interest is 2% and the term structure of interest rates will remain flat over the 3-year horizon
• Collateral and exposure values will remain stable over the life of the contract
Given the information and the assumptions above, what is the correct estimate for the credit valuation adjustment for this position?
选项:
A.AUD 0.140 million
B.AUD 0.863 million
C.AUD 1.291 million
D.AUD 2.514 million
解释:
To derive the credit valuation adjustment (CVA), we use the standard formula:
Where (at any time t),
• The discount factor (DFt) is determined from the risk-free rate of 2%; and
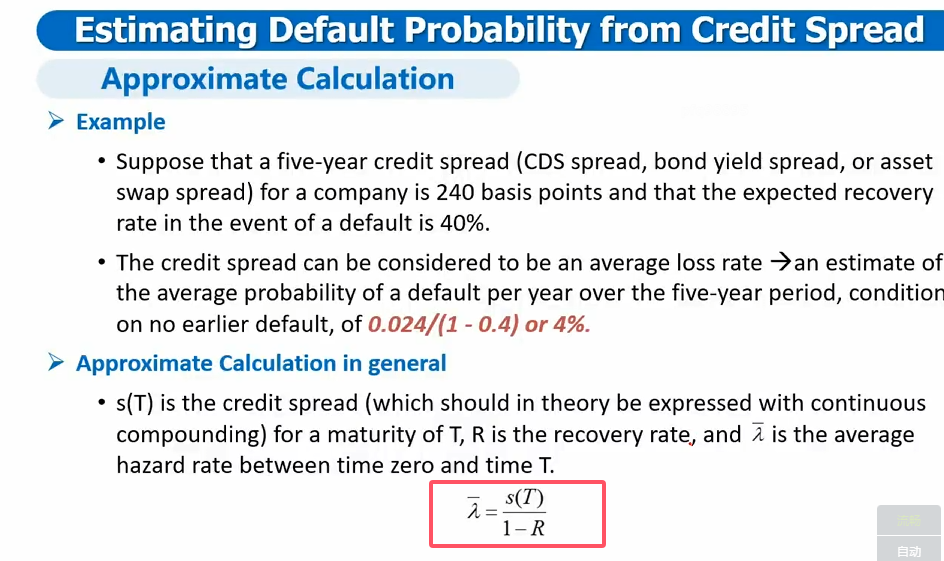
• The hazard rate = Spread/(1 – RR) = (180/10,000)/(1 – 0.85) = 12% (true for years 2 and 3);
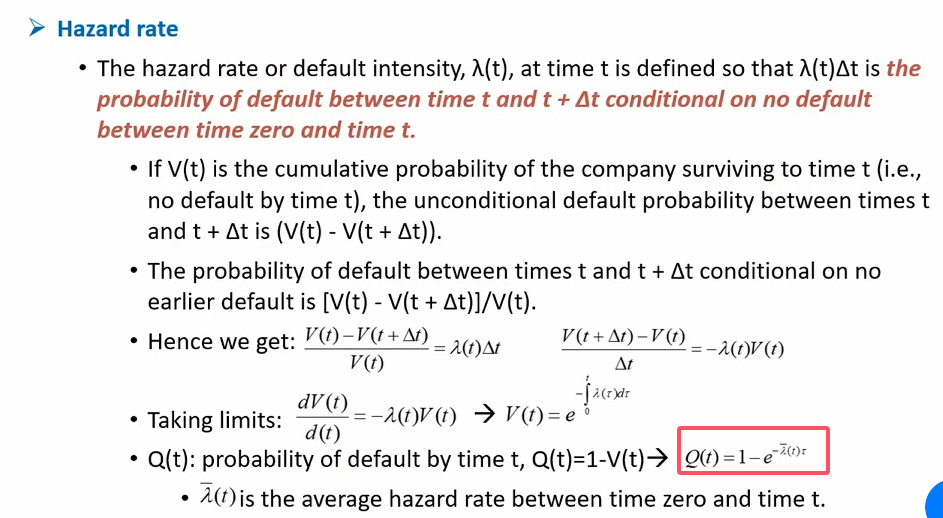
• The probability of default is derived from its relationship with the constant hazard rate (λ) ,
PD(t)=1-exp(-λt).
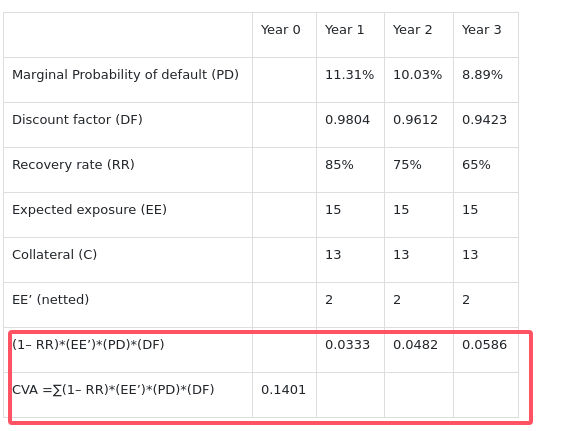
For instance, PD(1)=1-exp(-0.12*1)=11.31% (Marginal probability (PD1))
PD(2)=1-exp(-0.12*2) = 21.34%; Marginal probability (PD2)=21.34%-11.31%=10.03%
PD(3)=1-exp(-0.12*3) = 30.23%; Marginal probability (PD3)=30.23%-21.34%=8.89%
• Collateral amounts of AUD 13 million for year 2 and AUD 13 million for year 3 are considered.
Hence, the rest of the derivation becomes:
(Expected Exposure, Collateral, and CVA in AUD million)

这道题具体流程能否写一下