NO.PZ2022123001000126
问题如下:
The samples are drawn independently and both
populations are assumed to be normally distributed Using the above data, an
analyst is trying to test the null hypothesis that the population variances are
equal (H0: σ12=σ22) against the
alternative hypothesis that the variances are not equal (Ha: σ12≠σ22) at the 5% level of significance.
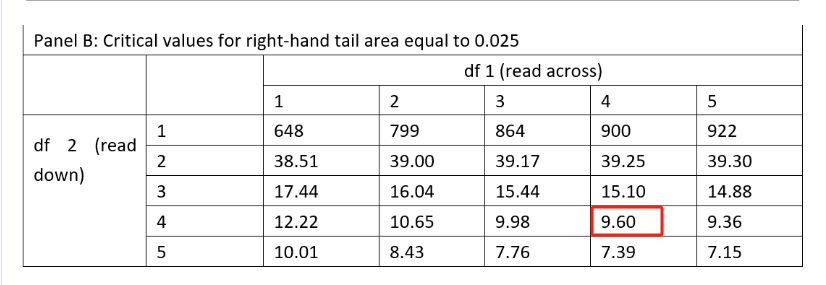
The table of the F-distribution is provided below.
Which of the
following statements is most appropriate? The critical value is:
选项:
A.6.39 and reject the
null.
9.60 and do not
reject the null
7.15and do not reject
the null
解释:
B is correct. The
test statistic makes use of the F-distribution and is the ratio of the
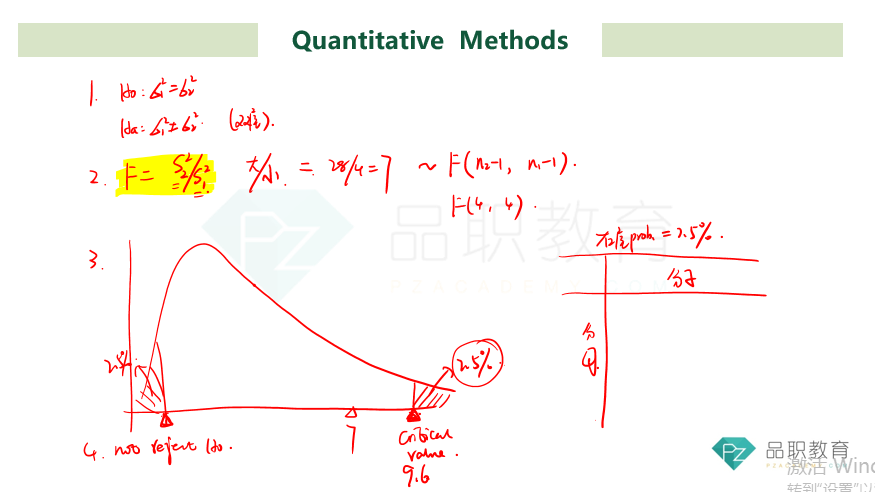
variances, with the larger variance in the numerator. The test statistic is F =
S22/ S12 = 28/4
= 7. The degrees of freedom are 4 by 4. As it is a two-tailed test, the correct
critical value at a=5% is 9.60(Panel B). As the test statistic is less than the
critical value(i.e.,7<9.60), the null hypothesis cannot be rejected.
A is incorrect.
It uses 4 by 4 degrees of freedom and does not divide the given alpha by two as
is appropriate for a two-tailed test.
C is incorrect. It uses the 5 by 5 critical
value (i.e.,5 degrees of freedom) rather than correctly using the 4 by 4 value
(i.e,4 degrees of freedom).
我记得之前说F分布只有右边的拒绝域,所以这里不用0.05/2? 还是我记错了?