NO.PZ2022010501000005
问题如下:
A European equity composite contains three portfolios whose cash flow weighting factors are as follows.
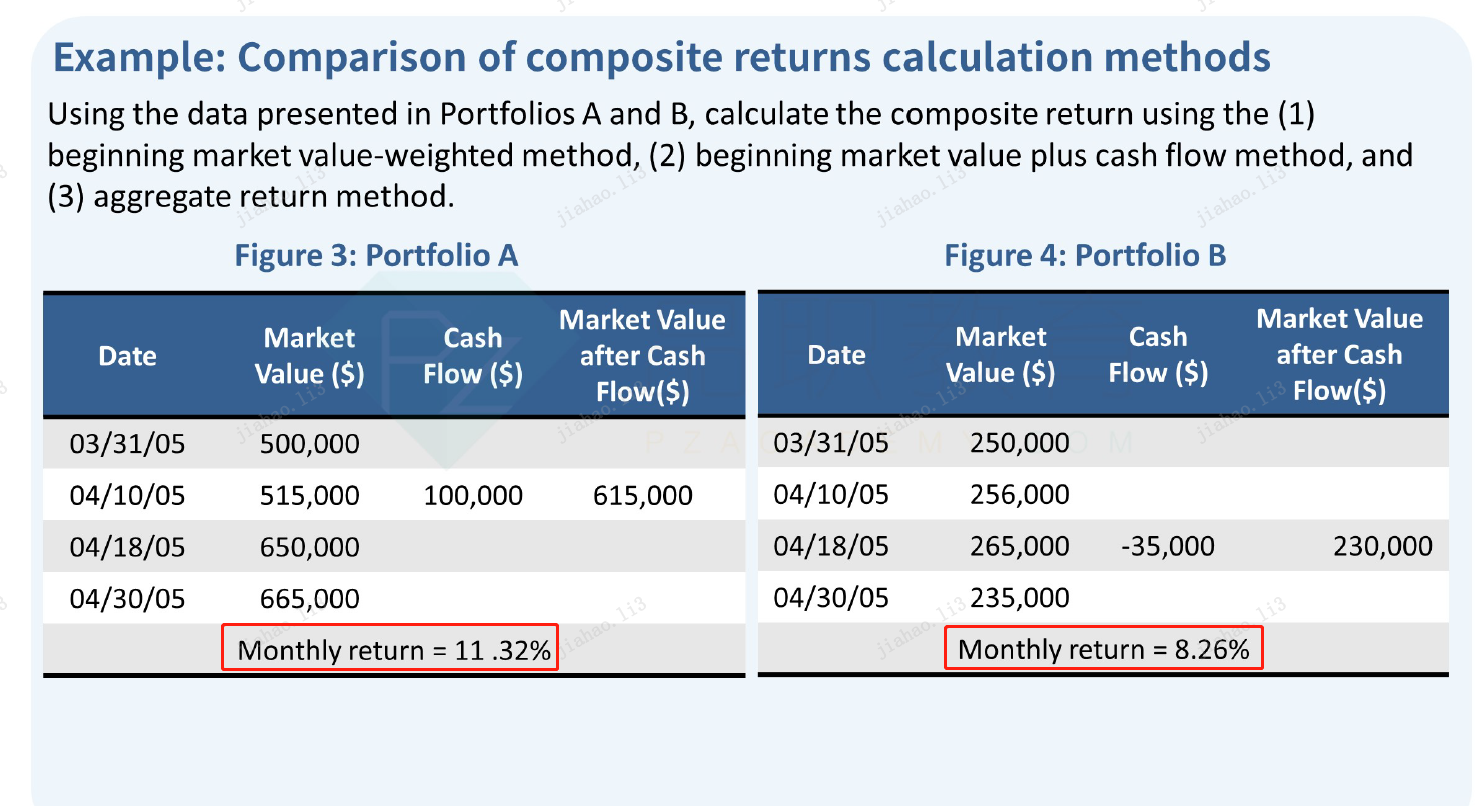
A Calculate the returns of Portfolio A, Portfolio B, and Portfolio C for the month of August using Modified Dietz formula.
B Calculate the August composite return by asset-weighting the individual portfolio returns using beginning-of- period values.
C Calculate the August composite return by asset- weighting the individual portfolio returns using a method that reflects both beginning-of-period values and external cash flows.
选项:
解释:
A Portfolio returns:
B To calculate the composite return based on beginning assets, first determine the percentage of beginning composite assets represented by each portfolio; then determine the weighted-average return for the month:
Beginning composite assets = 74.9 + 127.6 + 110.4 = 312.9
Portfolio A = 74.9/312.9 = 0.239 = 23.9%
Portfolio B = 127.6/312.9 = 0.408 = 40.8%
Portfolio C = 110.4/312.9 = 0.353 = 35.3%
C To calculate the composite return based on beginning assets plus cash flows, first use the denominator of the Modified Dietz formula to determine the percentage of total beginning assets plus weighted cash flows represented by each portfolio, and then calculate the weighted-average return:
Beginning composite assets + Weighted cash flows = [74.9 + (7.5 × 0.613)] + [127.6 + (−15 × 0.742) + (−5 × 0.387)] + [110.4 + (15 × 0.387)] = 79.5 + 114.535 + 116.205 = 310.24
Portfolio A = 79.5/310.24 = 0.256 = 25.6%
Portfolio B = 114.535/310.24 = 0.369 = 36.9%
Portfolio C = 116.205/310.24 = 0.375 = 37.5%
The Aggregate Return method is calculated by summing beginning assets and intra- period external cash flows, treating the entire composite as though it were a single portfolio and then computing the return directly with the Modified Dietz formula.
问题A里算出来的三个portfolio的return,不都是用modified Dietz method算出来的么?为什么默认这个值就可以用作beginning-of-values method和beginning-of-period values plus external cash flows的分子呢?
我能理解B和C是在计算分母加权平均时与A不同,但从逻辑上来说,分子也应该用相应的算法呀(比如答案里问题C的第二种解法,就和分母的逻辑是一致的),虽然从计算结果来看,问题C的两种算法恰好算出来的结果差异不大
我知道从现有已知条件来看,只有通过问题A这么一种rate of return可得,但万一考试的时候有别的return rate呢?或者有别的条件让你另算问题B的rate of return,算出来呢?问题C的两种算法算出来的rate of return不一样,我觉得只有第二种,严格按照beginning-of-period values plus external cash flows方法计算才是对的
另外从书上例题来看,两个portfolio的monthly return已经是给定的了(虽然我也没弄明白这两个return是怎么算出来的),直接代入计算即可,我觉得在这里没法作为参考