NO.PZ2018122701000049
问题如下:
A portfolio consists of options on Microsoft and AT&T. The options on Microsoft have a delta of 1000, and the options on AT&T have a delta of 20000. The Microsoft share price is $120, and the AT&T share price is $30. Assuming that the daily volatility of Microsoft is 2% and the daily volatility of AT&T is 1% and the correlation between the daily changes is 0.3, the 5-day 95% VaR is
选项:
A.
26193
B.
25193
C.
27193
D.
24193
解释:
A is correct.
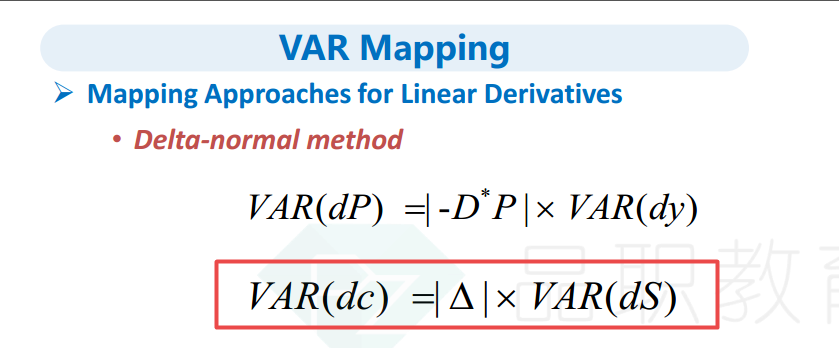
考点:Mapping to Option Position
解析:VaRMic= 1.65 × 2% × 120 × 1000 = 3960
VaRAT&T= 1.65 × 1% × 30 × 20000=9900
能详细讲下原理吗?两个var的组合求法在哪讲的呀?还有就是为啥是乘根号5啊?