NO.PZ202208220100000508
问题如下:
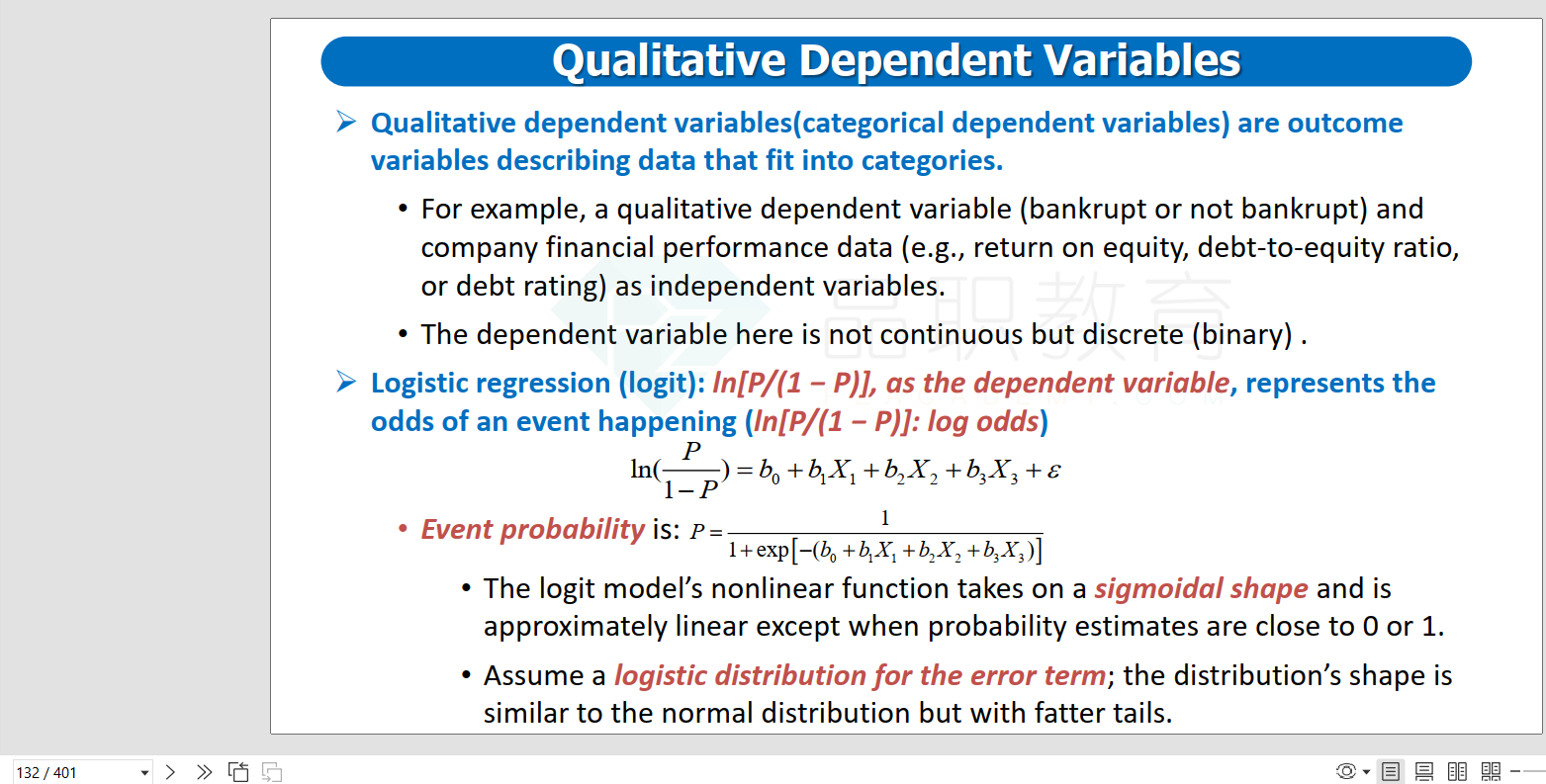
Based on the output for Logistic Regression 1 in the table below, which of the following alternatives is closest to the probability that any ETF will be a winning
fund?
选项:
A.6.75%
B.5.96%
C.5.67%
解释:
C is correct. We calculate the probability that an that an ETF will be a winning
fund by using the variable estimates and the average values of the independent
variables.
Using the equation for the probability, where we have seven independent
variables,
Using the mean values and coefffcient estimates of the independent variables, the
probability of the average ETF being a winner is
This implies that for an ETF with the average values of the independent variables,
there is a 5.67% probability that it will be a winning ETF.
请问这个公式哪里有讲过?
exp在公式里是什么意思?