NO.PZ2023062701000002
问题如下:
An analyst has developed a test to identify a certain disease. Research shows that 85% of people with the disease test positive, while 95% of healthy individuals test negative. It is estimated that 4% of the population has the disease. If a person tests positive, what is the posterior probability that they actually have the disease?
选项:
A.
41.46%
B.
85%
C.
50%
解释:
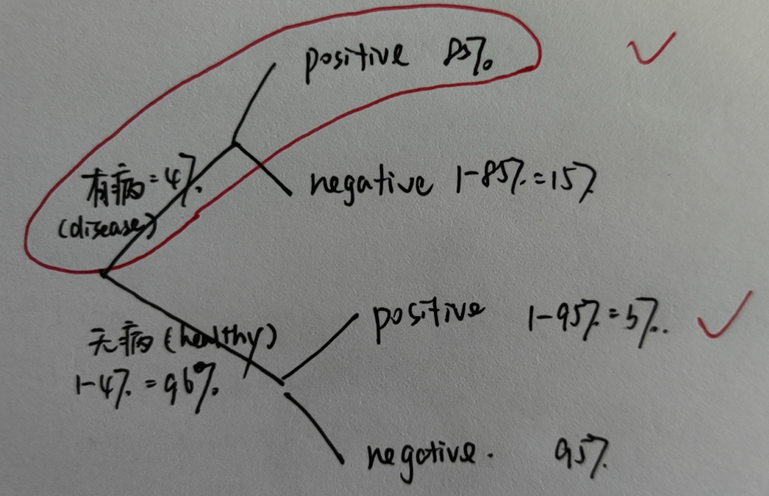
Let event A represent the person having the disease, and event B represent the person testing positive for the disease.
We are given:
P(A) = 0.04 (the probability of a person having the disease)
P(B|A) = 0.85 (the probability of testing positive given the person has the disease)
P(not A) = 1 - P(A) = 1 - 0.04 = 0.96 (the probability of a person not having the disease)
P(B|not A) = 0.05 (the probability of testing positive given the person does not have the disease)
We need to find P(A|B), the posterior probability that a person has the disease given that they tested positive.
By applying Bayes’ theorem: P(A|B) = (P(B|A) * P(A)) / P(B) P(B) can be calculated using the law of total probability:
P(B) = P(B|A) * P(A) + P(B|not A) * P(not A)
Substituting the given values:
P(B) = (0.85 * 0.04) + (0.05 * 0.96) = 0.034 + 0.048 = 0.082
Now, calculating P(A|B) using Bayes’ theorem:
P(A|B) = (P(B|A) * P(A)) / P(B) = (0.85 * 0.04) / 0.082 = 0.034 / 0.082 = 0.4146
The posterior probability that a person has the disease given that they tested positive is approximately 41.46%.
這道題應該要怎麼做?4%有什麼用