NO.PZ2022062760000012
问题如下:
An analyst is examining a portfolio that consists of 1,000 subprime mortgages and 600 prime mortgages. Of
the subprime mortgages, 200 are late on their payments. Of the prime mortgages, 48 are late on their
payments. If the analyst randomly selects a mortgage from the portfolio and it is currently late on its
payments, what is the probability that it is a subprime mortgage?
选项:
A.60%
B.67%
C.75%
D.81%
解释:
中文解析:
首先计算任何一个mortgage late的概率
P(Mortgage is late) = (200+48)/(1000+600) = 15.5%
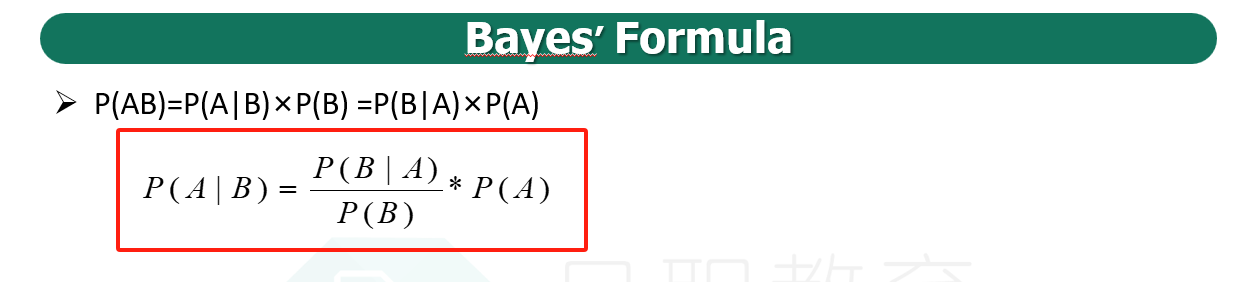
利用贝叶斯公式:
P(Subprime mortgage | Mortgage is late) = P(Subprime mortgage and late)/P(Mortgage is late).
已知:
P(Subprime mortgage and late) = 200/1600 = 12.5%;
得:
P(Subprime mortgage | Mortgage is late) = 12.5% / 15.5% = 0.81 = 81%
In order to solve this conditional probability question, first calculate the probability that any one mortgage in the portfolio is late.
This is: P(Mortgage is late) = (200+48)/(1000+600) = 15.5%.
Next, use the conditional probability relationship as follows:
P(Subprime mortgage | Mortgage is late) = P(Subprime mortgage and late)/P(Mortgage is late).
Since P(Subprime mortgage and late) = 200/1600 = 12.5%;
P(Subprime mortgage | Mortgage is late) = 12.5% / 15.5% = 0.81 = 81%.
Hence the probability that a random late mortgage selected from this portfolio turns out
to be subprime is 81%.
这道题能用画图,两步骤的方式来做么