此题如何计算callable bond 用exhibit1&2???

选项:
A. 
B. 
C. 
解释:

发亮_品职助教 · 2018年06月09日
这道题求含权债券价格和我们用二叉树求含权债券的做法是一模一样的。
只不过,我们二叉树里面见到的利率发展路径是这样的,见红框:

而本题的利率发展路径是这样的:

无论是哪种路径,求含权债券的价格本质上都是一样的:
都是将下一年的总现金流(包括债券价格和Coupon)折现到上一个node,得到上一个node的债券价值,看看上一个node的债券价值是否会触发行权价。
如果触发就调整债券价值至行权价,然后加上该node的coupon组成该node的现金流,继续往上一个node折。
只不过二叉树每一个树杈都是50%的权重;而本题的利率没有分叉,所以是100%的权重。
按题目给的信息:
Bond 4是一个maturity 3年,每年都可以用Par value行权的callable bond。
由于是每年都可以行权,所以每一年年末的时候(每一个Node),都要对比一下债券的价格是否会触发行权价。
需要用每一年的One-year forward rate,将下一年的债券现金流(债券的价值加上coupon)折现到本期。得到本期的债券价格(价值)。
从第三年年末开始,将第三年的总现金流(债券到期面额,加上第三年的Coupon)折现到第二年末,得到第二年年末的债券价值;用到的折现率是第二年末的One-year forward rate:

发现第二年末的债券价格为100.1952大于行权价100,这是callable bond,所以第二年末触发行权,将债券价格调整到100。
然后将第二年末调整后的债券价格100加上本年Coupon,组成第二年年的总现金流,折现到第一年末,用到的折现率是第一年末开始的One-year forward rate:
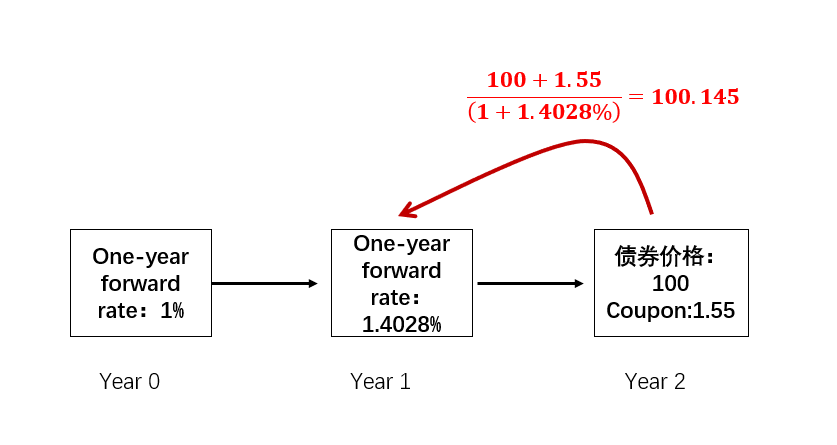
同理,callable bond,折现回来的价格发现触发行权价,将第一年末的债券价格调整到100,加上第一年的coupon,就能得到第一年的总现金流,把其往现在时刻折现,折现率是现在起的one-year forward rate:

所以,callable bond的现值是100.545
有些题目会让求某个node的债券价值,所以把发生在该node之后的现金流折现到该node就好,折现回来后不用加该node的Coupon。
本题并不是常见的二叉树,所以意味着one-year forward rate的权重是100%,当出现二叉树时,每一个叉的权重是50%,所以折现回来求每个节点现值时要乘权重。
NO.PZ201712110200000304 问题如下 Baseon the information in Exhibit 1 anExhibit 2, the value of the embeeoption in Bon4 is closest to: A.nil. B.0.1906. C.0.8789. C is correct. Bon4 is a callable bon Value of issuer call option = Value of straight bon– Value of callable bon The value of the straight bonmcalculateusing the spot rates or the one-yeforwarrates.Value of option-free (straight) bonwith a 1.55% coupon using spot rates:1.55/(1.0100)1 + 1.55/(1.012012)2 + 101.55/(1.012515)3 = 100.8789.The value of a callable bon(par) with no call protection periocannot excee100, thprior higher the bonwoulcalle The value of the call option = 100.8789 – 100 = 0.8789. 这道题V-straight_bon一开始是用SPOT RATE,从第三期往前折算(二叉树方法,只是不用分叉计算),发现出来结果是101.1742和答案100.8788不一样。然后我用forwarrate算一次发现结果就是100.8788,由此联想到,请问二叉树中各期利率是不是forwarrate? 我一直理解为SPOT RATE
NO.PZ201712110200000304 问题如下 Baseon the information in Exhibit 1 anExhibit 2, the value of the embeeoption in Bon4 is closest to: A.nil. B.0.1906. C.0.8789. C is correct. Bon4 is a callable bon Value of issuer call option = Value of straight bon– Value of callable bon The value of the straight bonmcalculateusing the spot rates or the one-yeforwarrates.Value of option-free (straight) bonwith a 1.55% coupon using spot rates:1.55/(1.0100)1 + 1.55/(1.012012)2 + 101.55/(1.012515)3 = 100.8789.The value of a callable bon(par) with no call protection periocannot excee100, thprior higher the bonwoulcalle The value of the call option = 100.8789 – 100 = 0.8789. callable bon价格有上限,不能超过100,所以,callable bonvalue应该是100,这句话怎么理解呢
NO.PZ201712110200000304 问题如下 Baseon the information in Exhibit 1 anExhibit 2, the value of the embeeoption in Bon4 is closest to: A.nil. B.0.1906. C.0.8789. C is correct. Bon4 is a callable bon Value of issuer call option = Value of straight bon– Value of callable bon The value of the straight bonmcalculateusing the spot rates or the one-yeforwarrates.Value of option-free (straight) bonwith a 1.55% coupon using spot rates:1.55/(1.0100)1 + 1.55/(1.012012)2 + 101.55/(1.012515)3 = 100.8789.The value of a callable bon(par) with no call protection periocannot excee100, thprior higher the bonwoulcalle The value of the call option = 100.8789 – 100 = 0.8789. embeoption bon以不考虑路径,用forwarrate求价值吗。。。
NO.PZ201712110200000304 问题如下 Baseon the information in Exhibit 1 anExhibit 2, the value of the embeeoption in Bon4 is closest to: A.nil. B.0.1906. C.0.8789. C is correct. Bon4 is a callable bon Value of issuer call option = Value of straight bon– Value of callable bon The value of the straight bonmcalculateusing the spot rates or the one-yeforwarrates.Value of option-free (straight) bonwith a 1.55% coupon using spot rates:1.55/(1.0100)1 + 1.55/(1.012012)2 + 101.55/(1.012515)3 = 100.8789.The value of a callable bon(par) with no call protection periocannot excee100, thprior higher the bonwoulcalle The value of the call option = 100.8789 – 100 = 0.8789. 但是我的疑惑是每次折现用什么数据,答案这里是spot rate进行折现,我却用了额one-yeforwar行折现,就是每次不知道用哪个数字合理?
NO.PZ201712110200000304 问题如下 Baseon the information in Exhibit 1 anExhibit 2, the value of the embeeoption in Bon4 is closest to: A.nil. B.0.1906. C.0.8789. C is correct. Bon4 is a callable bon Value of issuer call option = Value of straight bon– Value of callable bon The value of the straight bonmcalculateusing the spot rates or the one-yeforwarrates.Value of option-free (straight) bonwith a 1.55% coupon using spot rates:1.55/(1.0100)1 + 1.55/(1.012012)2 + 101.55/(1.012515)3 = 100.8789.The value of a callable bon(par) with no call protection periocannot excee100, thprior higher the bonwoulcalle The value of the call option = 100.8789 – 100 = 0.8789. The value of a callable bon(par) with no call protection periocannot excee100, thprior higher the bonwoulcalle为什么不会是小于100呢?没有赎回时间限制的callable bon什么一定价值是100呢?