NO.PZ2023040502000043
问题如下:
The analyst decides to do
additional analysis by first-differencing the data and running anew regression:
yt = b0 + b1yt–1 + εt,
where yt = xt – xt–1.
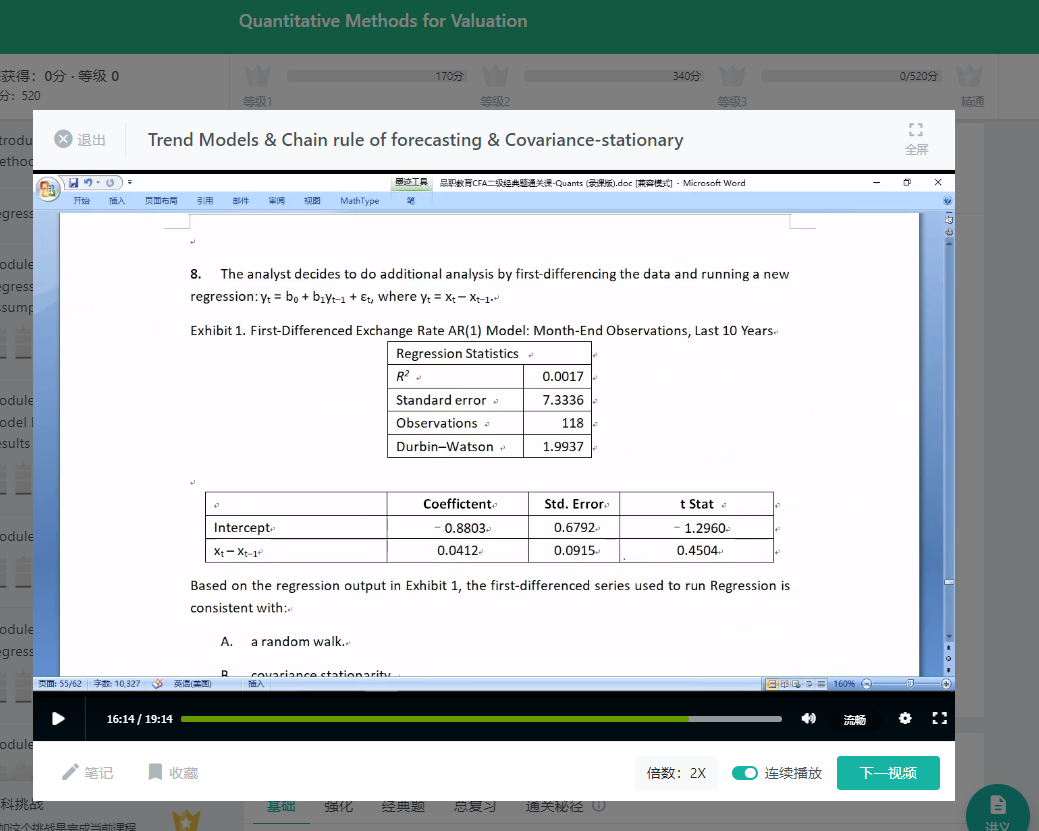
Exhibit 1. First-Differenced Exchange Rate AR(1)
Model: Month-End Observations, Last 10 Years
Based on the regression output in Exhibit 1, the first-differenced
series used to run Regression is consistent with:
选项:
A.a random walk
covariance stationarity
a random walk with drift
解释:
The critical t-statistic at a 5% confidence level is
1.98. As a result, neither the intercept nor the coefficient on the first lag
of the first-differenced exchange rate in Regression differs significantly from
zero. Also, the residual autocorrelations do not differ significantly from
zero. As a result, Regression can be reduced to yt = εt
with a mean-reverting level of b0/(1 – b1) = 0/1 =
0.Therefore, the variance of yt in each period is Var(εt)
= σ2. The fact that the residuals are not autocorrelated is
consistent with the covariance of the times series, with itself being constant
and finite at different lags. Because the variance and the mean of yt
are constant and finite in each period, we can also conclude that yt
is covariance stationary.
答案没看懂,可以讲解下吗