NO.PZ2015120204000015
问题如下:
Based on past research, Hansen selects the following independent variables to predict IPO initial returns:
Underwriter rank = 1–10, where 10 is highest rank
Pre-offer price adjustment (Expressed as a decimal) = (Offer price – Initial filing price)/Initial filing price
Offer size ($ millions) = Shares sold × Offer price
Fraction retained (Expressed as a decimal) = Fraction of total company shares retained by insiders
He also believes that for each 1 percent increase in pre-offer price adjustment, the initial return will increase by less than 0.5 percent, holding other variables constant. Hansen wishes to test this hypothesis at the 0.05 level of significance.
Hansen collects a sample of 1,725 recent IPOs for his regression model.
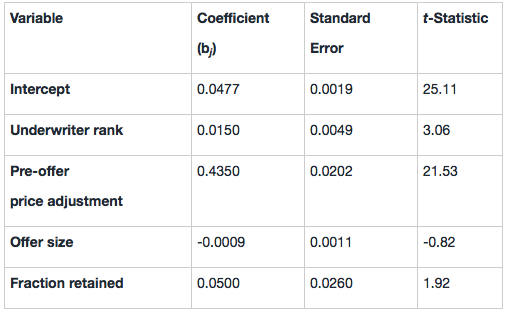
\Hansen’s Regression Results Dependent Variable: IPO Initial Return (Expressed in Decimal Form, i.e., 1% = 0.01)
Selected Values for the t-Distribution (df = ∞)

The most appropriate null hypothesis and the most appropriate conclusion regarding Hansen’s belief about the magnitude of the initial return relative to that of the pre-offer price adjustment (reflected by the coefficient bj) are:
选项:
解释:
C is correct.
C To test Hansen’s belief about the direction and magnitude of the initial return, the test should be a one-tailed test. The alternative hypothesis is H1: , and the null hypothesis is H0: . The correct test statistic is: t = (0.435-0.50)/0.0202 = -3.22, and the critical value of the t-statistic for a one-tailed test at the 0.05 level is -1.645. The test statistic is significant, and the null hypothesis can be rejected at the 0.05 level of significance.
为什么直接用-3.22和-1.645相比,而不是用-3.22和0.4350-1.645*0.0202相比,得到的结论是fall to reject H0



