四个问题解题思路与讲义88页不一致，不明白区别。-有问必答-品职教育专注CFA ESG FRM CPA 考研等财经培训课程

四个问题解题思路与讲义88页不一致，不明白区别。

NO.PZ2020010304000035

问题如下：

An experiment yields the following data:

It is hypothesized that the data comes from a uniform ditribution, U(0, b).

a. Calculate the sample mean and variance.

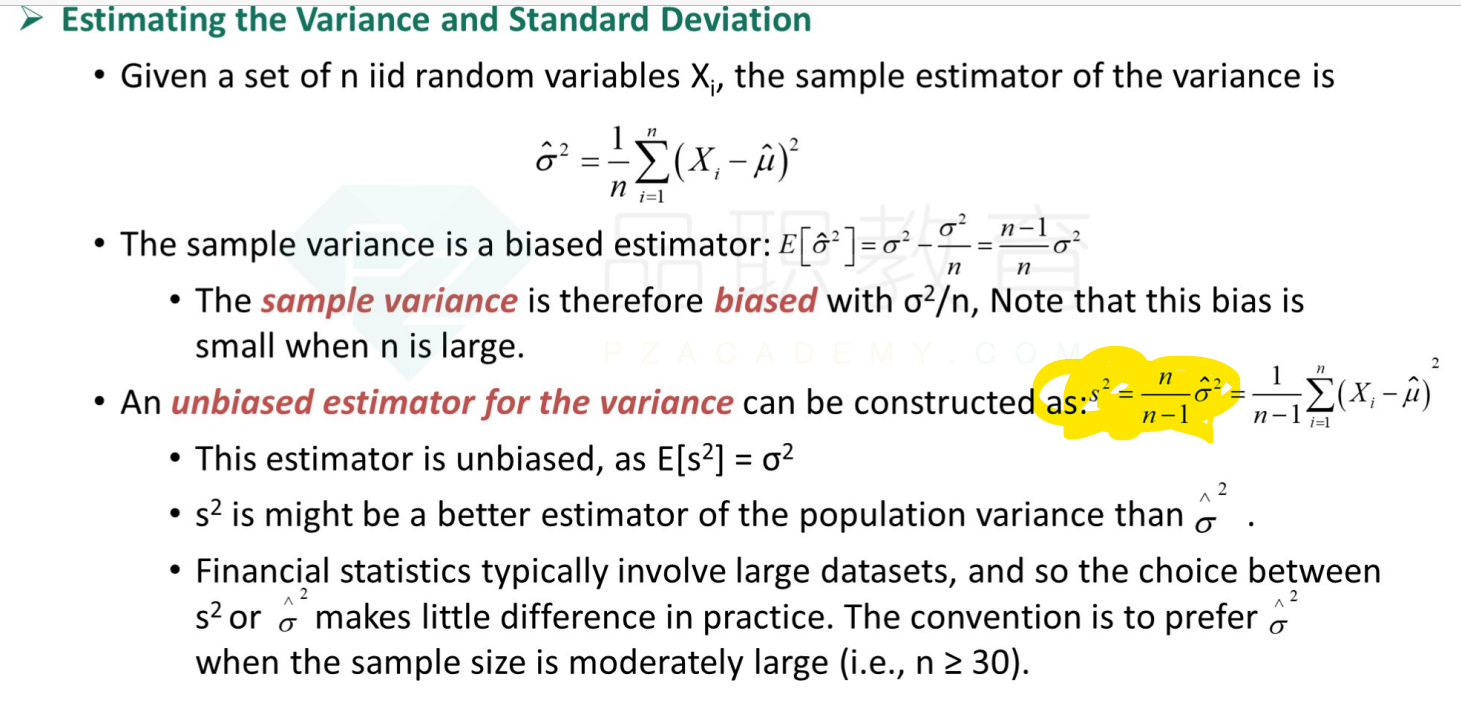
b. What are the unbiased estimators of the mean and variance?

c. Calculate the b in U(0, b) using the formula for the mean of a uniform distribution and the value of the unbiased sample mean found in part b.

d. Calculate the b in U(0, b) using the formula for the variance of a uniform distribution and the value of the unbiased sample variance found in part b.

解释：

a. Use the standard formual to get the sample variance(here, n=15)

$\mu = n^{-1}\sum_{i=1}^{n}X_i =0.39$

$\sigma^2 = n^{-1}\sum_{i=1}^{n}{(X_i-\mu)}^2 =0.08$

b.The sample mean is already unbiased.

For the variance:

$s^2=n\sigma^2/(n-1) =15*0.080/14=0.086$

c.The mean for a U(a,b) distribution is given as:

μ=(a+b)/2

0.385=(0+b)/2

b=0.77

d. The variance for a U(a,b) distribution is given as:

$\sigma^2=(b-a)^2/12$

$0.086=b^2/12$

$b=1.016$

样本均值应等于A加B然后除以2；无偏均值就是该均值。样本方差等于B减去A 平方然后除以12。解题公式不知道什么意思。最后两问不知道在说什么。均匀分布到底应该怎么计算？谢谢。尤其是最后两个问题在说什么。感觉均匀分布前提和适用场景特别不清楚。烦请详细说明，谢谢

嗨，爱思考的PZer你好：

同学你好，

这题就是简单的带公式，一点也不复杂，只要知道正确的公式代入数据就能得出答案：

a问求这组数据的样本均值和样本方差，均值很简单就是所有数据相加之和除个数，等于0.3853，约等于0.39。

样本方差=每个数据减去均值之后进行平方，加总这些平方之后除n-1，也就是14，得出方差=0.08

b求的是总体方差的无偏估计（Unbiased estimator），我们一般用样本方差（sample variance）的变形，作为总体方差的无偏估计：

unbiased estimator， s^2=σ^2 * n /(n−1)=15∗0.080/14=0.086

公式在讲义131页，如下图：

cd问求的是是均匀分布的均值和方差，这个可以参考基础班讲义P88

c问已知分布是U(0, b)，利用均值求b，用到的是第一个公式，a=0

均值=(a+b)/2

0.385=(0+b)/2

b=0.77

d问已知分布是U(0, b)，利用方差求b，用到的是第二个公式，a=0

方差=(b−a)^2/12

0.086=(b-0)^2/12

b=1.016

----------------------------------------------
虽然现在很辛苦，但努力过的感觉真的很好，加油！

四个问题解题思路与讲义88页不一致，不明白区别。

1 个答案

1

0

311

相关问题