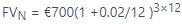NO.PZ2017092702000019
问题如下:
A client invests €20,000 in a four-year certificate of deposit (CD) that annually pays interest of 3.5%. The annual CD interest payments are automatically reinvested in a separate savings account at a stated annual interest rate of 2% compounded monthly. At maturity, the value of the combined asset is closest to:
选项:
A.€21,670.
B.€22,890.
C.€22,950.
解释:
B is correct,
as the following cash flows show:

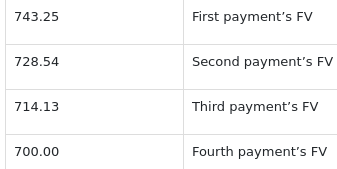
The four annual interest payments are based on the CD’s 3.5% annual rate. The first payment grows at 2.0% compounded monthly for three years (where FV is future value): FVN = €700(1 +0.02/12 )3×12 FVN = 743.25 The second payment grows at 2.0% compounded monthly for two years: FVN = €700(1 +0.02/12 )2×12 FVN = 728.54 The third payment grows at 2.0% compounded monthly for one year: FVN = €700(1 +0.02/12 )1×12 FVN=714.13
The fourth payment is paid at the end of Year 4. Its future value is €700. The sum of all future value payments is as follows:

根据EAR的公式:(1+2%/12)^12=1+EAR,得到EAR=2.0184%。
然后:
1. 首先按照年金的方式来计算利息再投资的终值:N=4,I/Y=2.0184,PV=0,PMT=700,CPT FV=-2885.9208;其中700是20,000每年按照3.5%产生的利息,I/Y是转化的EAR,因为利息(PMT)的频率也是一年一付的。
2. 然后把期初的这20,000再加回去,得到22,885.92。(和选项B有写出入是因为四舍五入的问题,但不影响选出答案)
老师您好,这道题逻辑还是没弄清楚,烦请详细推导一下。还有可以直接用公式计算吗?公式怎么列呢?EAR在什么情况下需要计算,什么情况下可以直接用期利率,这类题型每回都不太懂怎么计算,谢谢!