开发者:上海品职教育科技有限公司 隐私政策详情

应用版本:4.2.11(IOS)｜3.2.5(安卓)APP下载

返回

胖婷肥周 · 2022年07月12日

老师这个解题步骤错在哪里？

  1. CFA I
  2. Quantitative

NO.PZ2015120604000147

问题如下：

Here is a table discribing sample statistics from two bonds' rate of return which are both normally distributed over the past decades. If an investor is considering whether the mean of bond B is greater than 26%,

which of the following conclusion is least appropriate (significant level=5%) ?

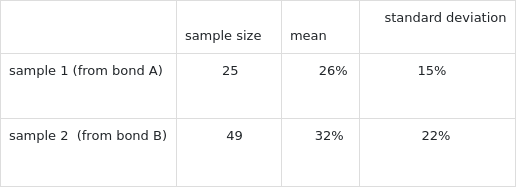
选项：

A.

It is approptiate to use the Z-test.

B.

The mean of bond B is not significant greater than 26%.

C.

It is a one-tailed test.

解释：

B is correct.

It is appropraite to ues the one-tailed Z-test because the sample size of bond B is bigger than 30.

The null hypothesis is: H0: μ ≤ 26%

The test statistic:


α= 5% indicates the critical value is equal to ±1.65.

Because 1.91>1.65, therefore, we should reject the null hypothesis.

我算出了1.91，然后用1.91去跟置信区间的关键值做比较。这个关键值，我看解题里面是1.65，但是5%是单尾的吖，为什么还是1.65呢？双尾±1.65吖。所以，我找的是t分布，单尾5%的，值是1.68。然后，1.91是大于1.68的，也就是在他的j Object的区间里，所以拒绝原假设，应该所以 H0≤26%是错误的。那么就是均值＞26%是正确呢，这样不是应该选择B吗？

添加评论
1 个答案

星星_品职助教 · 2022年07月13日

同学你好，

1）1.65对应的是正态分布下，单尾面积为5%时的情况；

2）本题也可以使用t检验的关键值，结论同样是test statistic＞critical value，所以要拒绝原假设。

根据题干，可得出原假设为H0：μ≤26%，

故最终结论为the mean of bond B is significant greater than 26%

  • 1

    回答

  • 0

    关注

  • 291

    浏览
相关问题

NO.PZ2015120604000147问题如下 Here is a table scribing sample statistifrom two bon' rate of return whiare both normally stributeover the past cas. If investor is consiring whether the meof bonB is greater th26%,whiof the following conclusion is least appropriate (significant level=5%) ?A.It is appropriate to use the Z-test.B.The meof bonB is not significant greater th26%.C.It is a one-tailetest. B is correct.It is appropraite to ues the one-taileZ-test because the sample size of bonB is bigger th30.The null hypothesis is: H0: μ ≤ 26%The test statistiα= 5% incates the criticvalue is equto ±1.65. Because 1.91 1.65, therefore, we shoulrejethe null hypothesis. 单尾的5% α 是1.65，双尾是1.96 对吗老师

2024-10-14 11:35 1 · 回答

NO.PZ2015120604000147 问题如下 Here is a table scribing sample statistifrom two bon' rate of return whiare both normally stributeover the past cas. If investor is consiring whether the meof bonB is greater th26%,whiof the following conclusion is least appropriate (significant level=5%) ? A.It is appropriate to use the Z-test. B.The meof bonB is not significant greater th26%. C.It is a one-tailetest. B is correct.It is appropraite to ues the one-taileZ-test because the sample size of bonB is bigger th30.The null hypothesis is: H0: μ ≤ 26%The test statistiα= 5% incates the criticvalue is equto ±1.65. Because 1.91 1.65, therefore, we shoulrejethe null hypothesis. 怎么确定原假设方向是小于？根据是否大于，怎么确定原假设和备择假设，，怎么分析

2024-07-22 18:07 1 · 回答

NO.PZ2015120604000147 问题如下 Here is a table scribing sample statistifrom two bon' rate of return whiare both normally stributeover the past cas. If investor is consiring whether the meof bonB is greater th26%,whiof the following conclusion is least appropriate (significant level=5%) ? A.It is appropriate to use the Z-test. B.The meof bonB is not significant greater th26%. C.It is a one-tailetest. B is correct.It is appropraite to ues the one-taileZ-test because the sample size of bonB is bigger th30.The null hypothesis is: H0: μ ≤ 26%The test statistiα= 5% incates the criticvalue is equto ±1.65. Because 1.91 1.65, therefore, we shoulrejethe null hypothesis.

2024-06-03 12:29 1 · 回答

NO.PZ2015120604000147 问题如下 Here is a table scribing sample statistifrom two bon' rate of return whiare both normally stributeover the past cas. If investor is consiring whether the meof bonB is greater th26%,whiof the following conclusion is least appropriate (significant level=5%) ? A.It is appropriate to use the Z-test. B.The meof bonB is not significant greater th26%. C.It is a one-tailetest. B is correct.It is appropraite to ues the one-taileZ-test because the sample size of bonB is bigger th30.The null hypothesis is: H0: μ ≤ 26%The test statistiα= 5% incates the criticvalue is equto ±1.65. Because 1.91 1.65, therefore, we shoulrejethe null hypothesis. 当落在阴影的时候，rejeH0，接受Ha,则表达为is significantily fferent from H0么？

2024-05-16 00:09 1 · 回答

NO.PZ2015120604000147问题如下Here is a table scribing sample statistifrom two bon' rate of return whiare both normally stributeover the past cas. If investor is consiring whether the meof bonB is greater th26%,whiof the following conclusion is least appropriate (significant level=5%) ?A.It is appropriate to use the Z-test.B.The meof bonB is not significant greater th26%.C.It is a one-tailetest. B is correct.It is appropraite to ues the one-taileZ-test because the sample size of bonB is bigger th30.The null hypothesis is: H0: μ ≤ 26%The test statistiα= 5% incates the criticvalue is equto ±1.65. Because 1.91 1.65, therefore, we shoulrejethe null hypothesis. 请问对于这道题大样本的情况，计算出来t统计量后查对应的5%的criticvalue是不是既可以查z分布表也可以查t分布表

2024-03-13 15:27 1 · 回答